5 Key Answers for Graphing Motion Worksheet
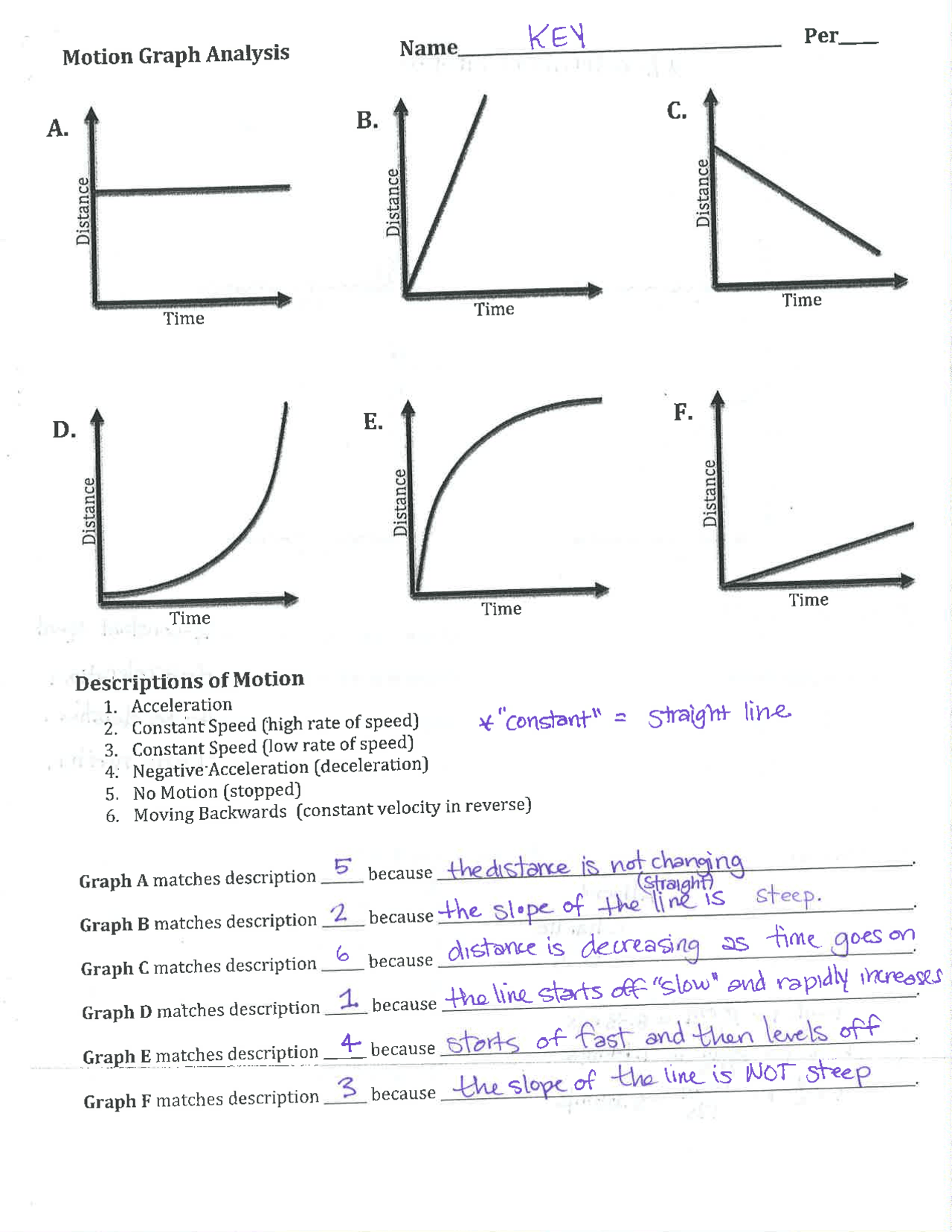
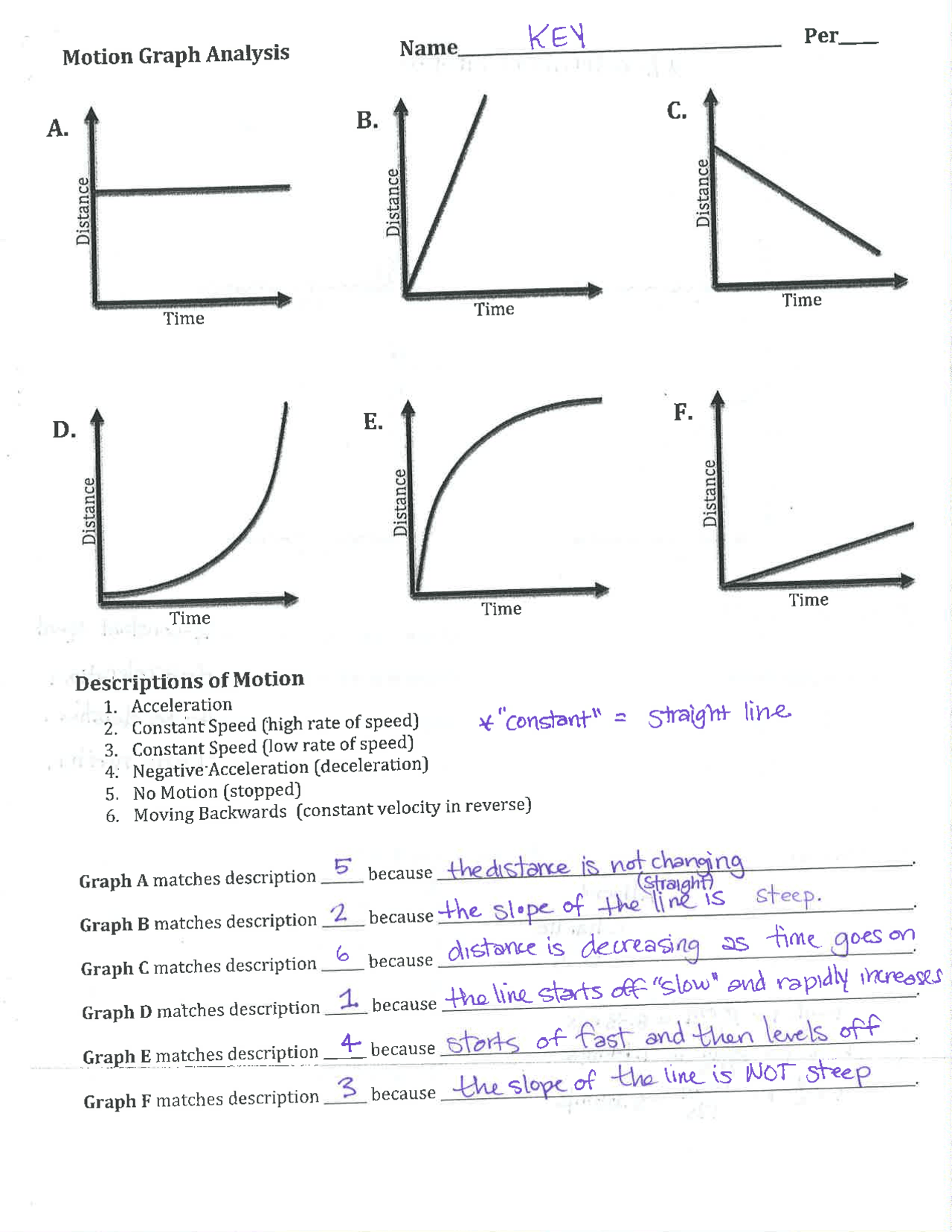
In the study of physics, understanding motion is fundamental. One of the most effective ways to visualize and interpret motion is through graphing. A graphing motion worksheet typically includes exercises that require students to plot, interpret, and analyze various types of motion graphs, such as position-time and velocity-time graphs. Here are five key answers and insights you might find helpful while tackling such a worksheet:
1. Understanding Position-Time Graphs


- Constant Velocity: A straight line with a constant slope indicates motion at a constant velocity. The slope gives the velocity.
- Rest: A horizontal line represents an object at rest, as the position isn't changing over time.
- Acceleration: A curved line suggests acceleration. The slope at any point gives the instantaneous velocity.
2. Analyzing Velocity-Time Graphs


- Constant Velocity: A horizontal line indicates constant velocity, where the area under the curve gives the total displacement.
- Acceleration: A sloped line means the object is accelerating. The slope represents the acceleration.
- Displacement: The area under the graph provides the displacement. For non-uniform acceleration, sum up the areas for different segments.
3. Interpreting Negative Values on Graphs

- Position-Time: A negative slope means the object is moving back towards the initial point or even past it.
- Velocity-Time: Negative velocity indicates motion in the opposite direction. A negative acceleration indicates deceleration if moving forward or acceleration if moving backward.
4. Calculating Acceleration from Graphs

To find acceleration from a velocity-time graph:
- Use the formula a = (Δv/Δt) where Δv is the change in velocity and Δt is the time interval over which this change happens.
📝 Note: For uniform acceleration, this is straightforward, but for varying acceleration, you might need to average over small intervals or integrate if using calculus.
5. Analyzing Multiple Segments
When dealing with motion that changes character (e.g., constant velocity followed by acceleration):
- Segment Analysis: Break the motion into segments where velocity or acceleration is consistent. Analyze each part separately for velocities, displacements, and accelerations.
- Total Displacement: Sum up the displacements from each segment to find the total displacement.
- Average Velocity: Use v_avg = Δd/Δt where Δd is the total displacement and Δt is the total time.
Mastering these techniques not only helps in completing graphing motion worksheets but also in understanding real-world phenomena. Each graph provides a snapshot of motion, enabling us to predict, interpret, and design physical scenarios. Whether it's planning the trajectory of a rocket or simply understanding how speed limits work in traffic, these foundational skills are invaluable. Remember, the key to success with motion graphs is to relate each part of the graph to the underlying physics principles - velocity, acceleration, and displacement.
What does a flat line on a velocity-time graph represent?
+
A flat line on a velocity-time graph represents constant velocity. The object is moving at a steady speed without acceleration.
How can you find distance traveled from a velocity-time graph?

+
You can find the distance traveled by calculating the area under the velocity-time curve. For rectangles, it’s simple; for other shapes, break it into smaller segments or use calculus for exact results.
Is it possible for an object to have zero velocity but not zero acceleration?

+
Yes, at the peak or trough of a trajectory, an object can have zero velocity but still be accelerating (decelerating) as it changes direction.