Compound Shapes Worksheet Answers: Complete Guide

Learning how to work with compound shapes is an essential part of mastering basic geometry. Compound shapes, also known as composite shapes, are figures made up of two or more simple geometric shapes. Understanding these shapes allows students to solve more complex problems involving area, perimeter, and volume. This guide provides a comprehensive overview of compound shapes, their properties, and how to tackle common worksheet questions effectively.
Understanding Compound Shapes

Compound shapes can range from the combination of squares and rectangles to more intricate figures involving circles, triangles, and other polygons. Here are the key points to understand:
- Definition: A shape made up of two or more basic shapes.
- Common Shapes Involved: Rectangles, squares, circles, triangles, parallelograms, and trapezoids.
- Application: Used in real-world contexts like floor plans, landscape designs, and structural engineering.
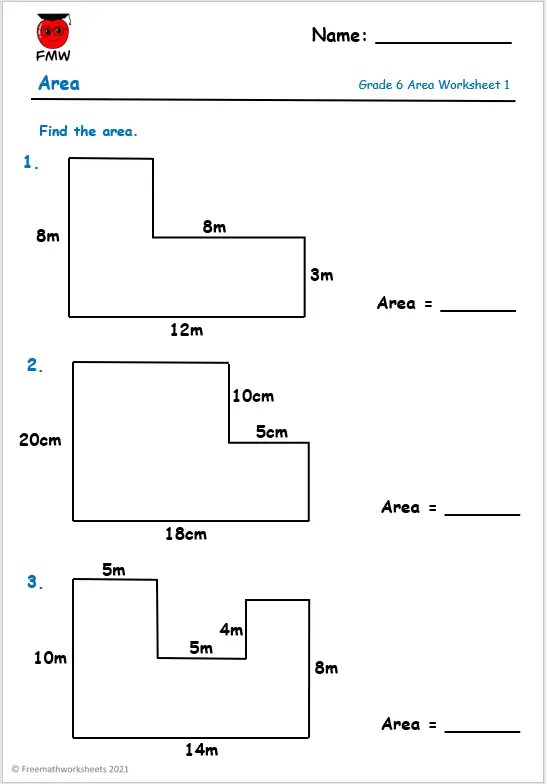
Calculating Area of Compound Shapes

Calculating the area of compound shapes involves several steps:
- Break Down the Shape: Identify and separate the individual shapes making up the compound figure.
- Calculate Each Part: Find the area of each component shape individually using standard formulas.
- Add or Subtract Areas: Depending on the arrangement, sum or subtract these areas to get the total or the required part's area.
Here are some formulas for common shapes:
| Shape | Area Formula |
|---|---|
| Rectangle | A = l × w |
| Triangle | A = 0.5 × b × h |
| Circle | A = πr² |

Example: If a compound shape consists of a rectangle with dimensions 5m by 4m attached to a triangle with a base of 3m and height of 2m:
- Area of Rectangle = 5m × 4m = 20m²
- Area of Triangle = 0.5 × 3m × 2m = 3m²
- Total Area of Compound Shape = 20m² + 3m² = 23m²
🔎 Note: Always check the units of measurement to ensure consistency.
Dealing with Overlapping Shapes

When shapes overlap:
- Calculate the area of each shape as if they did not overlap.
- Subtract the area of the overlapping part from the sum of individual areas.
Solving Perimeter Problems

The perimeter of compound shapes involves:
- Tracing the outer edge of the entire shape.
- Adding up all the external edges, ensuring not to count any shared edge twice.
Examples of Compound Shape Worksheets

Here are typical problems you might encounter:
Example 1: Area

Find the area of a compound shape formed by a semicircle sitting atop a rectangle:
- Rectangle dimensions: 10 cm (width) by 8 cm (length).
- Semicircle with radius 5 cm (half of rectangle’s width).
The area can be calculated as:
- Area of Rectangle = 10 cm × 8 cm = 80 cm²
- Area of Semicircle = (π × (5 cm)²) / 2 ≈ 39.27 cm²
- Total Area = 80 cm² + 39.27 cm² = 119.27 cm²
Example 2: Perimeter

Calculate the perimeter of a shape where a 5 cm side square is attached to the left side of a rectangle with dimensions 10 cm by 15 cm:
- Perimeter = 10 cm + 15 cm + (10 cm - 5 cm) + 15 cm + 5 cm = 40 cm
In this summary, we’ve covered how to break down compound shapes into simpler forms to calculate area and perimeter. We’ve looked at how overlapping shapes affect these calculations and provided examples to illustrate these concepts. Understanding and applying these principles will help you tackle any compound shape worksheet with confidence. Practice is key, so working through various problems will refine your geometric reasoning skills.
The FAQ section below addresses common questions to further clarify any lingering doubts or curiosities you might have.
How do I know which shapes make up a compound shape?

+
Observe the distinct parts of the shape. Look for straight lines, curves, and angles that suggest different basic geometric figures like rectangles, triangles, circles, etc.
What if the compound shape has overlapping regions?

+
Calculate the area of each individual shape as if there were no overlaps, then subtract the overlapping area to find the true area of the compound shape.
Can compound shapes have volume?

+
Yes, if the compound shape is a 3D object, it has volume. You’ll need to calculate the volumes of the individual solids and combine them accordingly.
Is there a trick to quickly assess compound shape areas?
+
Practice mental dissection of shapes. With enough practice, you can quickly identify common shapes within compound shapes, reducing the time needed to solve problems.