5 Simple Tricks to Master Angles in Triangles Worksheet
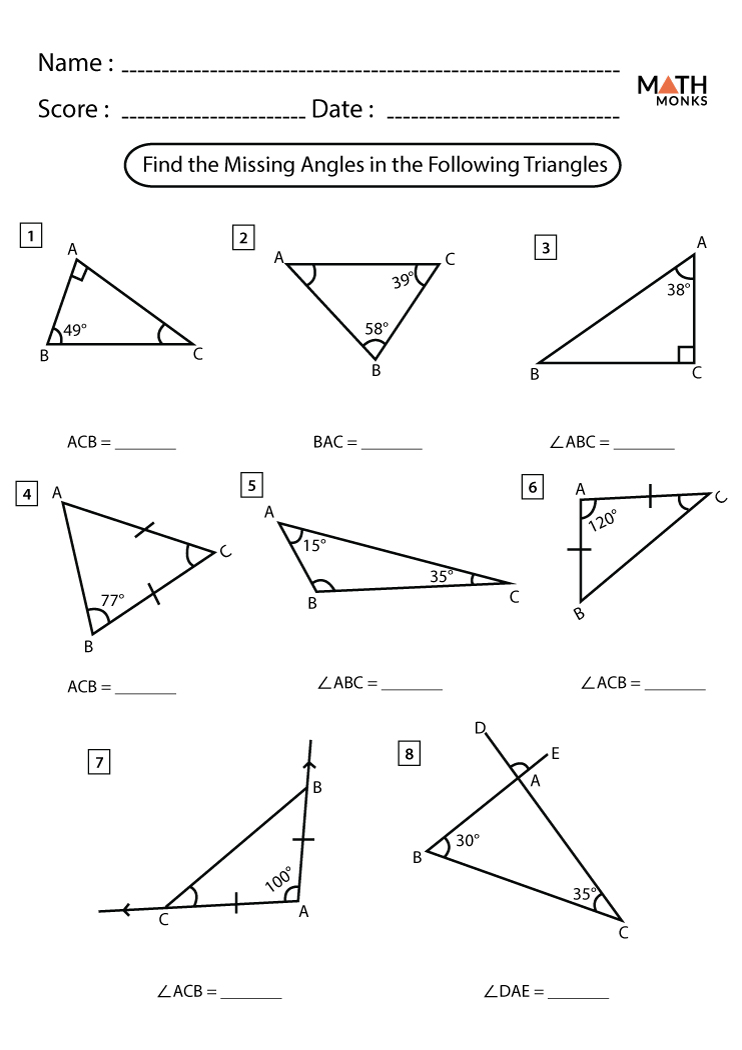
Triangles are foundational shapes in geometry, with applications ranging from structural engineering to art and design. Understanding angles in triangles is crucial for students and enthusiasts alike. Here's how you can master these concepts with simple yet effective tricks when working through angles in triangles worksheets:
1. Master the Basics with Sum of Angles

Begin with the fundamental principle:
- The sum of interior angles of any triangle equals 180 degrees.
This fact is invaluable for solving a myriad of triangle-related problems. If you’re working on a worksheet where only two angles are given, you can always calculate the third using the formula:
- Angle C = 180° - (Angle A + Angle B)
This technique ensures that no matter what form the problem takes, you have a reliable method to find missing angles.
2. Use Your Knowledge of Special Triangles

Equilateral, isosceles, and right triangles each have unique angle properties:
- Equilateral triangles: Each angle is exactly 60°.
- Isosceles triangles: Two sides and two angles are congruent. If you know one of the base angles, the other is the same.
- Right triangles: One angle is 90°, making the sum of the other two 90°.
Image: Equilateral, isosceles, and right triangles.
🔢 Note: Use these special triangle properties to your advantage when analyzing complex worksheets with multiple triangles.
3. Engage with Triangle Sum Properties

Beyond the basic sum of angles, there are properties that extend from this fundamental rule:
- Exterior angles theorem.
- Interior angles properties.
When confronted with exterior angles, remember:
- An exterior angle of a triangle is equal to the sum of the two non-adjacent interior angles.
By identifying these relationships, you can simplify the calculations required in your worksheet exercises.
4. Draw to Visualize

Often, visualization can be your best tool. Here’s how to draw to understand:
- Sketch out the triangle if not provided.
- Label all known information.
- Draw additional lines like medians or altitudes if they might help reveal geometric properties or simplify angle calculations.
Visualization aids in better understanding the relationships between angles and sides, making complex problems seem more manageable.
5. Understand Angle Relationships

Angles in triangles have various relationships:
- Vertically opposite angles.
- Complementary angles (summing to 90°).
- Supplementary angles (summing to 180°).
Knowing these can help when you’re asked to find angles that aren’t directly part of the triangle or when problems include additional lines intersecting the triangle.
⚙️ Note: Familiarize yourself with common angle relationships to solve problems more efficiently.
As you wrap up your journey through angles in triangles, remember that consistent practice with worksheets will enhance your geometrical intuition and problem-solving skills. By employing these tricks, you'll not only gain mastery over basic triangle angles but also develop an approach to tackle more complex geometric problems. Over time, the relationships and calculations will become second nature, turning once-challenging exercises into enjoyable puzzles.
What is the trick to finding the missing angle in a triangle?

+
The simplest trick is to use the triangle angle sum theorem. If you know two angles, subtract their sum from 180° to find the third angle.
How do I distinguish between different types of triangles?

+
Triangles are distinguished by their sides and angles. Here’s a quick reference:
- Equilateral: All sides and angles equal.
- Isosceles: Two sides/angles are equal.
- Scalene: All sides/angles different.
Can I use the sum of angles for all triangles, even irregular ones?

+
Yes, the sum of interior angles always equals 180°, regardless of the shape or irregularity of the triangle.
Why do we need to understand angles in triangles?

+
Understanding angles in triangles is essential for practical applications in construction, architecture, trigonometry, and many fields involving geometric shapes.
How do I check my work on triangle angle worksheets?

+
Double-check your work by ensuring all angles add up to 180°, use the exterior angle theorem where applicable, and verify relationships among angles as described.