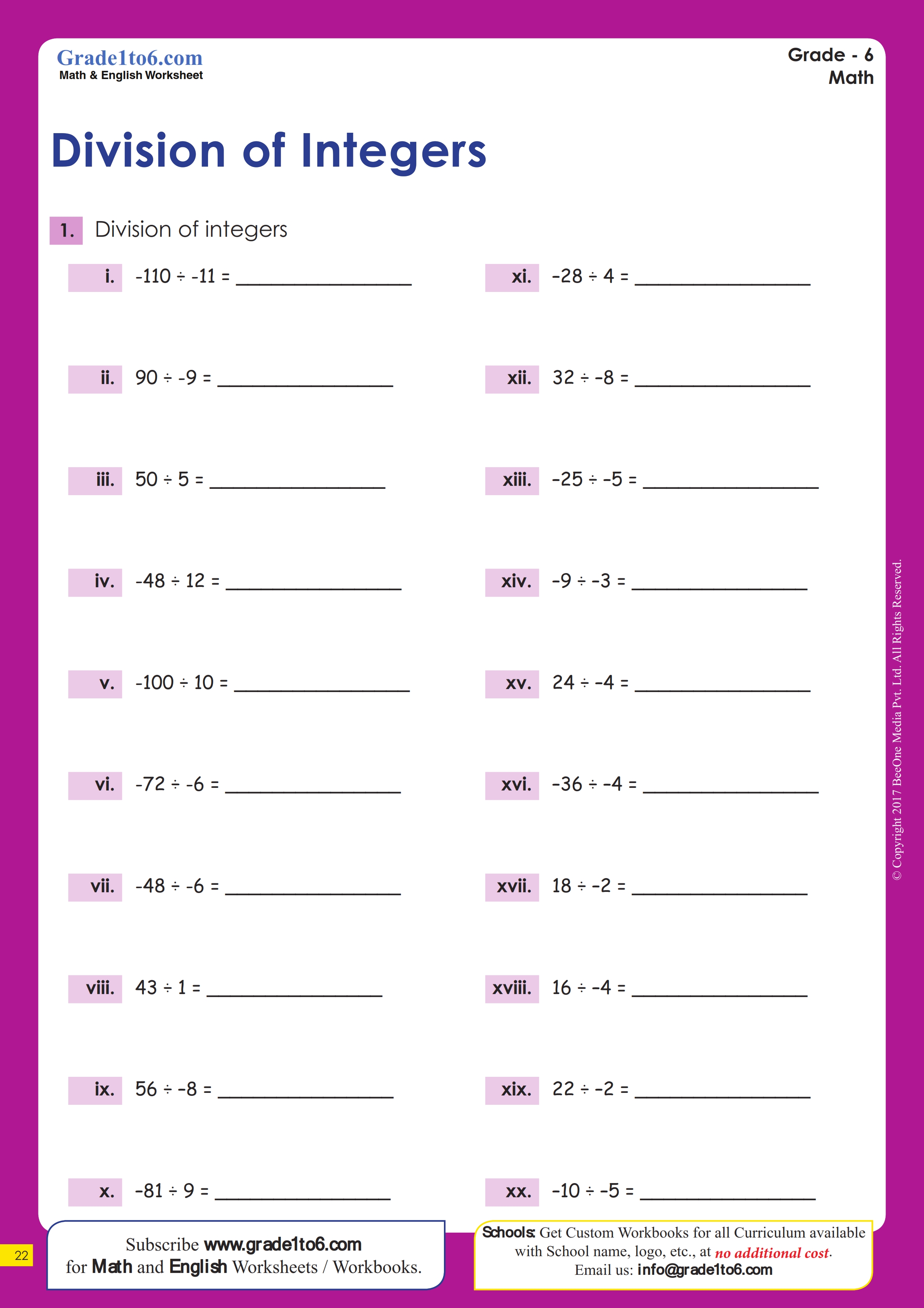
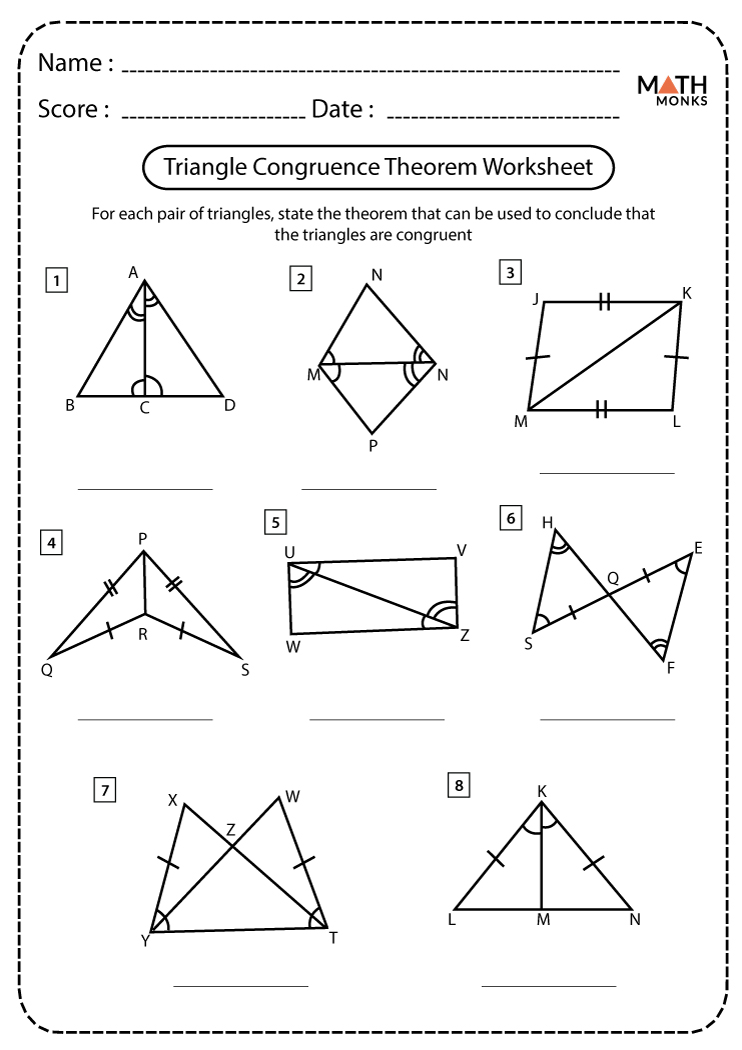
5 Key Angle Relationships You Should Know

Understanding angle relationships is crucial in geometry, as these relationships can greatly simplify solving complex problems involving angles. From basic angle pairs like supplementary and complementary angles to intricate ones like vertical and corresponding angles, each type has unique properties and rules. Here, we'll explore five key angle relationships you should know to ace your geometry tasks or just to appreciate the beauty of this branch of mathematics.

1. Supplementary Angles

Supplementary angles are two angles whose measures add up to 180 degrees. Imagine walking along a straight line; when you reach the end, you’ve completed 180 degrees. Here are the characteristics:

• They form a linear pair when placed adjacent to each other.
• Each angle complements the other to reach 180 degrees.
• Commonly found in angles formed by a straight line.

Examples include the angles of a linear pair or the interior angles on the same side of a transversal.

📌 Note: Supplementary angles don't need to be adjacent or touching, but when they are, they form what's known as a linear pair.

2. Complementary Angles

Complementary angles add up to a total of 90 degrees. Picture a right angle, like the corner of a room or the bend in your elbow when you make a right angle. Their properties include:

• They fit together to form a right angle.
• They are commonly used when bisecting angles.
• Can be non-adjacent but add up to 90 degrees when brought together.

📌 Note: Like supplementary angles, complementary angles don’t have to be adjacent, but when they are, they form a right angle.

3. Vertical Angles

Vertical angles, or opposite angles, are formed when two lines intersect. These angles have some intriguing properties:

• They are congruent (equal in measure).
• They are located diagonally opposite each other at the intersection point.
• Vertical angles are always equal regardless of the intersection’s position or angles’ sizes.

📌 Note: Vertical angles provide a quick method to find angles when intersecting lines are involved.

4. Corresponding Angles

When a transversal intersects two parallel lines, the angles formed in similar positions (same relative location) are called corresponding angles. Here are their key features:

• They are congruent if the lines are parallel.
• They are situated on the same side of the transversal.
• Corresponding angles can help confirm the parallelism of two lines.

📌 Note: When proving parallel lines, look for pairs of corresponding angles that are equal.

5. Alternate Interior Angles

Also formed by a transversal intersecting parallel lines, alternate interior angles are interior angles on opposite sides of the transversal:

• They are congruent when the lines are parallel.
• Lie between the two parallel lines but on opposite sides of the transversal.
• They form a “Z” shape if the lines are parallel.

📌 Note: Alternate interior angles are particularly useful for proving parallelism in a diagram.

By understanding these five key angle relationships, you open up a world of geometric problem-solving. These relationships don't just help with basic geometry tasks; they're foundational for more complex mathematical inquiries like trigonometry or even in the real-world applications of geometry in architecture and design. Mastering these concepts allows you to approach geometric problems with confidence, giving you the tools to dissect complex shapes into simpler components or to prove geometric theorems with elegance and clarity. With this knowledge, you can navigate the intricate world of angles, lines, and shapes with ease, enhancing both your analytical skills and your appreciation for the intricate beauty of geometry.