7 Essential Tips for Mastering Angle Geometry Worksheets

Understanding angles and their measurements is a cornerstone of geometry, integral not only for academic purposes but also for practical applications in various fields such as architecture, engineering, and design. Angle geometry worksheets provide an excellent opportunity to practice and master the concepts of angles. Here are seven essential tips to help you excel in working with these worksheets, making learning both enjoyable and effective.
Understand the Basics
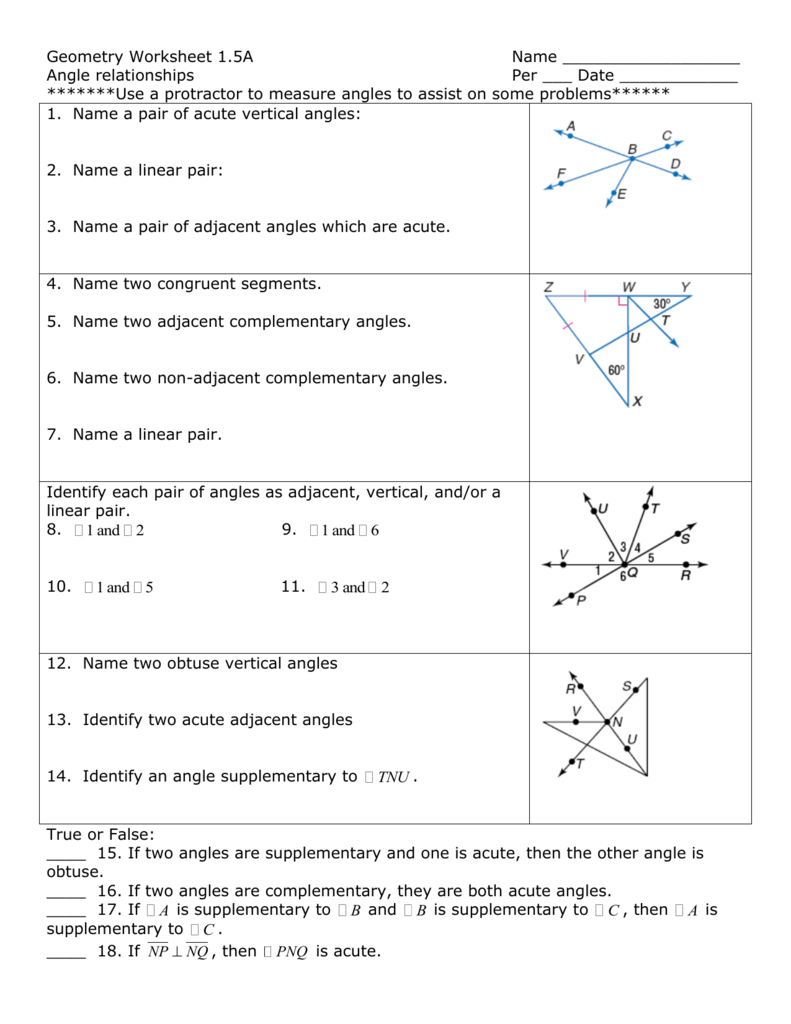
Before diving into the more complex aspects of angle geometry, it’s crucial to have a firm grasp on the fundamentals. Here are the key concepts to start with:
- Types of Angles: Acute, obtuse, right, and straight angles.
- Angle Properties: The sum of angles in a triangle, angles around a point, and the relationship between parallel lines and transversals.
- Complementary and Supplementary Angles: Understanding that complementary angles add up to 90 degrees and supplementary angles add to 180 degrees.
📌 Note: Familiarity with these basic concepts will significantly ease your journey through more advanced geometry problems.
Use Visual Aids

Geometry is inherently visual. Here’s how you can leverage this:
- Draw your angles or use provided diagrams. This visual representation helps in understanding the spatial relationships.
- Color-coding or marking angles with different symbols can make complex worksheets less intimidating.

Master Angle Labeling Techniques

Accurate labeling is vital for clarity in both your work and understanding. Here’s how you can excel:
- Always label vertices clearly. If a problem involves multiple angles at one vertex, label them as α (alpha), β (beta), etc.
- Label straight lines with the same letter and parallel lines with the same type of arrow.
🔍 Note: Proper labeling not only helps in solving problems but also makes your work verifiable and presentable.
Practice with Real-World Examples

Connecting academic exercises to real-life scenarios can deepen your understanding:
- Measure angles in your environment (e.g., the corners of a room, the angles formed by a ladder against a wall).
- Understand how angles affect designs, like in graphic design or structural engineering.
Develop Problem-Solving Strategies

A systematic approach to solving geometry problems can be beneficial:
| Strategy | Description |
|---|---|
| Identify the problem | Determine what you need to find (angle measurements, relationships, etc.) |
| List knowns | Note down any given measurements or relationships. |
| Visualize | Draw a sketch if one isn’t provided. |
| Apply formulae | Use angle properties or theorems like the exterior angle theorem. |

Regular Review and Practice

Consistency in practice is key:
- Set a daily or weekly goal for practicing angle geometry. Worksheets are perfect for this.
- Keep reviewing past worksheets to reinforce your learning and catch any overlooked mistakes.
Seek Feedback and Collaboration

Learning in isolation can limit perspective:
- Join study groups or online forums where you can discuss geometry problems with peers.
- Seek feedback from teachers or mentors to refine your problem-solving techniques.
In summary, mastering angle geometry worksheets involves understanding the basics, utilizing visual aids, adopting effective labeling practices, engaging with real-world examples, developing strategic problem-solving skills, committing to regular practice, and seeking collaborative learning opportunities. By following these tips, you'll not only improve your proficiency in angle geometry but also enhance your overall spatial reasoning and critical thinking abilities.
Why is understanding angles important in real-life applications?

+
Angles are crucial in various fields like architecture for designing structures, in engineering for ensuring stability, in navigation for determining directions, and in everyday activities like determining slopes for bike paths or angles for photography.
How can I make studying geometry more fun?

+
Integrate geometry into games or puzzles, use geometry apps or websites with interactive content, and relate geometry concepts to physical objects around you. Also, working on geometry projects can be both educational and entertaining.
What are some common mistakes students make in angle geometry?

+
Common errors include misidentifying angle types, not recognizing the relationships between angles (like supplementary and complementary angles), neglecting to label angles correctly, and failing to visualize the problem accurately.
How can I improve my spatial visualization skills for geometry?

+
Practice sketching geometric shapes, use 3D puzzles or modeling kits, play video games that require spatial reasoning, and engage in activities like origami that require folding and understanding space relations.
Is there a quick way to check my answers in geometry?

+
Use geometric properties to verify your answers. For instance, the sum of angles in a triangle should always be 180 degrees. You can also use online tools or apps that can instantly verify geometric solutions or provide step-by-step solutions.



