5 Must-Know Algebraic Limits for Math Enthusiasts

Mathematics, with its broad spectrum of topics, often leads enthusiasts down the fascinating path of calculus, where limits play a pivotal role. Understanding limits is crucial for mastering calculus, and algebra provides several fundamental limit examples that are both educational and intriguing. This post will delve into five must-know algebraic limits that every math enthusiast should familiarize themselves with. These limits not only illustrate the concept of approaching a specific value but also help in understanding the behavior of functions.
The Concept of Limits
Limits in mathematics define how a function behaves as its input approaches a certain value. They form the backbone of calculus, allowing us to:
- Determine continuity
- Calculate derivatives
- Analyze integrals
Limit 1: The Squeeze Theorem

The Squeeze Theorem, sometimes known as the Sandwich Theorem, is a powerful tool to find limits where direct substitution doesn't work.

- Problem: Find the limit of sin(x)/x as x approaches 0.
- Solution: By using the functions -|sin(x)| ≤ sin(x)/x ≤ |sin(x)| where -1 ≤ sin(x) ≤ 1, we can squeeze the function to find that sin(x)/x → 1.
Limit 2: Polynomials and Rational Functions

Polynomial and rational functions offer some of the most straightforward limits:
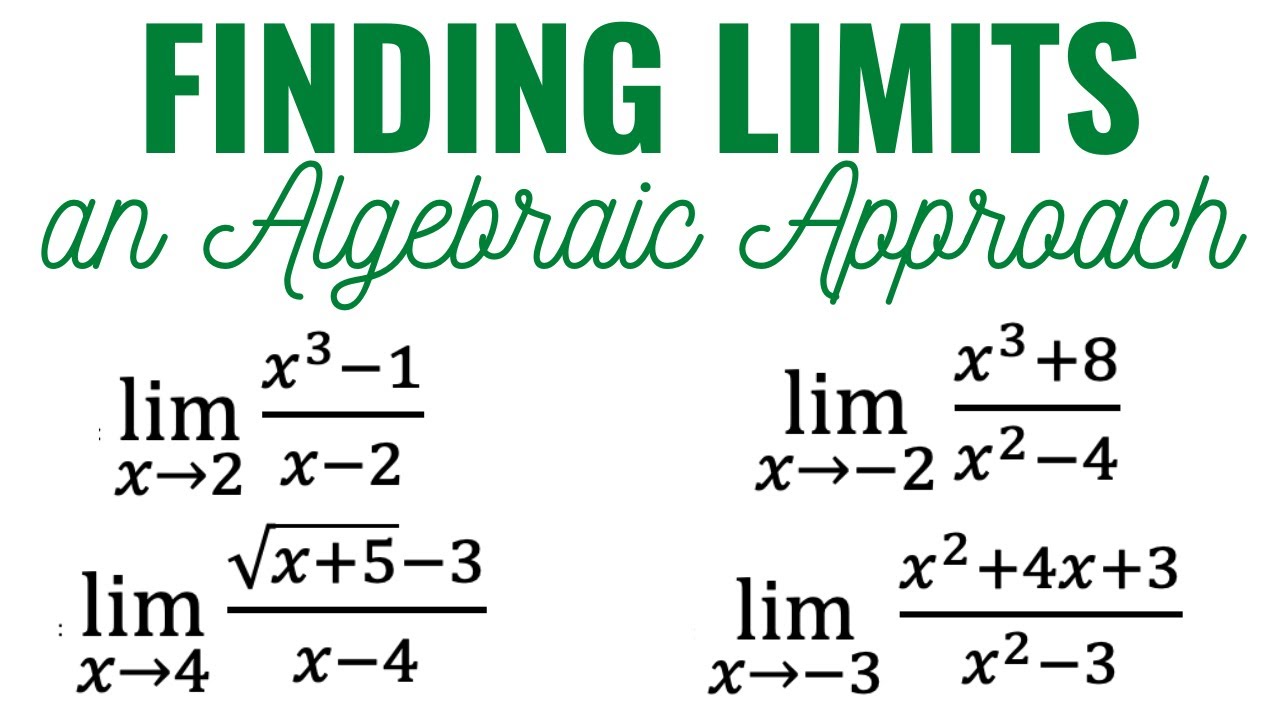
- Example: (x² + x - 6)/(x - 2) as x approaches 2.
- Solution: Factorizing, we get (x - 2)(x + 3)/(x - 2). Canceling out the common factor, the limit is (x + 3), which simplifies to 5.
Limit 3: Indeterminate Forms

When evaluating limits, we often encounter indeterminate forms like 0/0 or ∞/∞. Here's how to handle them:
- Example: (x - 2)/(x² - 4) as x approaches 2.
- Solution: Both the numerator and the denominator tend to zero, leading to an indeterminate form. Factorize and cancel to get 1/(x + 2), which gives a limit of 1/4.
Limit 4: Limits at Infinity

Understanding the behavior of functions as x tends to infinity is essential for studying growth rates and other phenomena:
- Example: lim (x → ∞) [x² - 3x + 2]/(x² + 2x + 1)
- Solution: Divide each term by x². As x tends to infinity, the limit simplifies to 1.
Limit 5: Trigonometric Limits

Trigonometric functions exhibit unique behaviors when approaching certain values:
- Example: lim (θ → 0) (sin(θ)/θ)
- Solution: This limit equals 1, as we discussed earlier with the Squeeze Theorem.
📘 Note: Recognizing when to use trigonometric identities or calculus techniques can simplify the process of finding limits.
Closing Thoughts
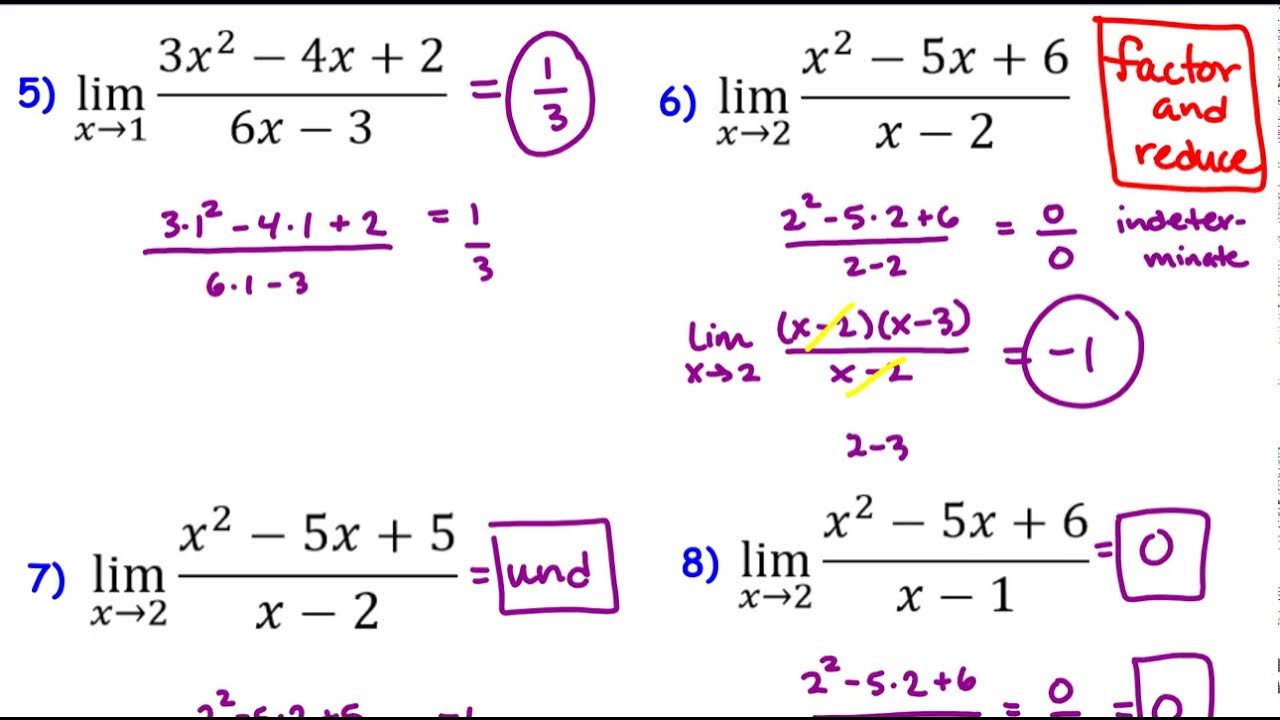
These five algebraic limits are just the tip of the iceberg. They provide a solid foundation for understanding how functions behave near specific points or as they tend towards infinity. Exploring limits through algebraic means not only deepens your mathematical intuition but also prepares you for more complex calculus topics like derivatives, integrals, and continuity.
What is the Squeeze Theorem used for?

+
The Squeeze Theorem is used to find limits of functions when direct substitution fails, by sandwiching the function between two others whose limits are known.
How do you handle an indeterminate form in limits?

+
Indeterminate forms can often be resolved by factoring, canceling common terms, or applying L’Hôpital’s Rule if the form persists.
Why are limits at infinity important?

+
Limits at infinity help us understand the long-term behavior of functions, which is vital in analyzing growth rates, decay, and stability in various fields of study.
Can you find a limit without calculus?

+
Yes, many limits can be determined using algebraic manipulation, direct substitution, or trigonometric identities without needing calculus techniques.
What’s the difference between limits approaching a finite value and approaching infinity?

+
When a limit approaches a finite value, the function tends towards a specific number as the input gets closer to a certain point. In contrast, when approaching infinity, the function’s value is assessed as the input variable grows without bound.