5 Simple Tips to Master Algebraic Expressions
In the vast landscape of mathematics, algebra stands as a cornerstone, a language where letters and symbols are used to represent numbers and operations. Algebraic expressions are fundamental to this language, and mastering them opens up a world of problem-solving and mathematical fluency. If you're looking to improve your grasp on algebraic expressions, here are five straightforward tips to guide you through this essential math journey.
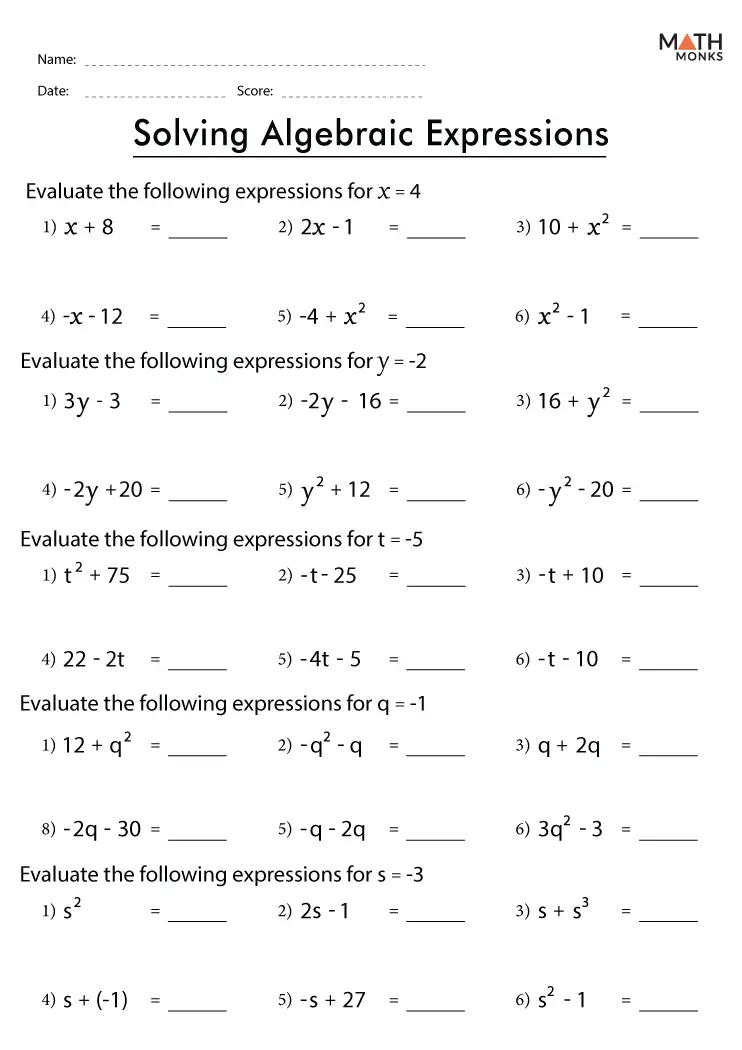
Tip #1: Understand the Basics

Before you dive deep into the manipulation of algebraic expressions, it’s crucial to ensure you’re solid on the fundamentals:
- Variables and Constants: Understand that a variable represents an unknown value or a quantity that can change, while constants are fixed values.
- Terms and Coefficients: A term in an algebraic expression can be a variable, a number, or a combination of both. The coefficient is the numerical factor of the term.
- Exponents: Learn how exponents affect the value of numbers and how to handle them in expressions.

⚠️ Note: Don’t skip over the basics. They are the foundation for everything else in algebra.
Tip #2: Practice Combining Like Terms

One of the most common operations you’ll perform on algebraic expressions is combining like terms. Here’s how to do it:
- Identify the like terms by looking for terms with the same variables raised to the same power.
- Add or subtract the coefficients of these like terms.
| Original Expression | Combined Expression |
|---|---|
| 2x + 5x + 3y | 7x + 3y |
| 9a - 3a + 2b - b | 6a + b |

📝 Note: Like terms must have the same variable and exponent; otherwise, they cannot be combined.
Tip #3: Master the Distributive Property

The distributive property is key in simplifying expressions:
- Multiply a term outside the parenthesis by each term inside the parenthesis.
- Combine like terms after distribution.
Example: 3(x + 2) = 3 * x + 3 * 2 = 3x + 6

🔧 Note: Don’t overlook the sign in front of each term when distributing.
Tip #4: Work with Exponents Confidently

Exponents play a pivotal role in algebraic expressions:
- Adding and Subtracting: Combine like terms with exponents; for example, x² + 3x² = 4x².
- Multiplying: When multiplying, add the exponents if the bases are the same.
- Dividing: When dividing, subtract the exponents if the bases are the same.

🏗️ Note: Exponents follow specific rules that differ from basic arithmetic.
Tip #5: Regularly Solve Equations

Turning expressions into equations and solving them will reinforce your understanding:
- Isolate the variable on one side of the equation.
- Perform inverse operations to isolate the variable.
To solve the equation 2x + 3 = 9, you would:
- Subtract 3 from both sides: 2x + 3 - 3 = 9 - 3 (which simplifies to 2x = 6)
- Divide both sides by 2: (2x) / 2 = 6 / 2 (which simplifies to x = 3)

🔎 Note: Solving equations helps you practice all aspects of algebraic manipulation.
Wrapping it up, by focusing on these five tips—understanding the basics, practicing combining like terms, mastering the distributive property, working confidently with exponents, and solving equations regularly—you'll significantly enhance your ability to handle and solve algebraic expressions. Remember, algebra is not just about following steps; it's about developing a mental toolkit that allows you to approach and solve problems in creative and effective ways. Keep practicing, stay curious, and you'll soon find that algebra isn't just a hurdle to overcome but a fascinating aspect of mathematics that empowers you in various aspects of life.
What are the key elements of an algebraic expression?

+
The key elements include variables, constants, coefficients, terms, and sometimes exponents or parentheses.
How do I simplify algebraic expressions?

+
Use the following steps to simplify expressions: - Combine like terms - Apply the distributive property - Handle exponents correctly
Why is understanding the distributive property important?

+
Understanding the distributive property allows you to expand and simplify expressions, which is crucial for solving equations and factoring.
What are some common mistakes when working with algebraic expressions?
+
Common mistakes include: - Incorrectly combining terms - Not handling negative signs properly - Forgetting the rules for exponents