10 Essential Algebra 2 Worksheets for Better Problem-Solving

Algebra 2 forms the backbone of advanced mathematics, where students delve deeper into the concepts they learned in Algebra 1, tackling more complex equations, functions, and abstract theories. Whether you're a student striving to master Algebra 2 or an educator looking for resources to better teach it, having a collection of well-designed worksheets can significantly enhance the learning experience. Here, we provide a comprehensive guide on 10 essential Algebra 2 worksheets that are instrumental for better problem-solving.
1. Rational Expressions Worksheet

Understanding rational expressions is pivotal in Algebra 2. This worksheet focuses on:
- Simplifying rational expressions.
- Multiplying and dividing rational expressions.
- Adding and subtracting rational expressions.
The worksheet should include a mix of simple and complex problems to ensure students can practice:
- Factoring techniques.
- Identifying common factors.
- Finding the least common denominator (LCD).
⚠️ Note: Complex fractions often require students to first express all terms with a common denominator before simplifying or operating.
2. Quadratic Functions Worksheet

Quadratic functions are an integral part of Algebra 2:
- Completing the square.
- Using the quadratic formula.
- Solving quadratic inequalities.
The worksheet might include:
- Problems requiring the use of the discriminant to determine the nature of solutions.
- Real-life applications where quadratic equations model a situation.
- Graphing quadratic functions to visualize the roots.
3. Logarithms and Exponential Functions Worksheet

Logarithms and exponential functions can be challenging:
- Converting between logarithmic and exponential forms.
- Solving exponential equations.
- Properties of logarithms (product, quotient, power rule).
Exercises should include:
- Word problems involving compound interest, population growth, etc.
- Problems requiring the use of change of base formula.
4. Complex Numbers Worksheet

Complex numbers are introduced in Algebra 2, providing a broader understanding of numbers:
- Operations with complex numbers.
- Graphing complex numbers.
- Using the imaginary unit i.
💡 Note: Complex numbers can simplify problems involving quadratic equations with negative discriminants.
5. Systems of Equations Worksheet
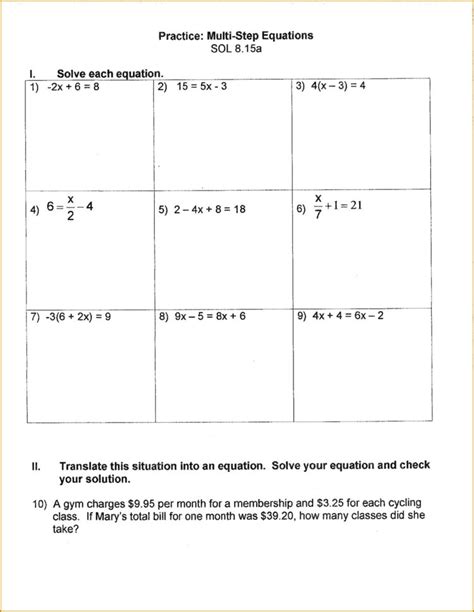
Solving systems of equations involves multiple strategies:
- Substitution method.
- Elimination (addition-subtraction) method.
- Graphing to find intersection points.
Worksheet examples should cover:
- Linear systems.
- Systems with quadratic equations.
- Word problems that require setting up and solving systems of equations.
6. Matrices and Determinants Worksheet

Matrices are powerful tools for handling systems of equations:
- Addition and subtraction of matrices.
- Multiplication of matrices.
- Determinants and inverses of matrices.
Include:
- Transformation of matrices.
- Using matrices to solve systems of linear equations.
7. Sequences and Series Worksheet

Sequences and series are key concepts:
- Arithmetic sequences and series.
- Geometric sequences and series.
- Infinite geometric series and their sums.
Practice problems should:
- Include finding the nth term of sequences.
- Summing series using formulas.
- Real-world applications like finance.
8. Conic Sections Worksheet

Conic sections open the door to fascinating geometry:
- Circles, ellipses, parabolas, and hyperbolas.
- Standard forms and transformations.
- Finding foci, vertices, and directrices.
Exercises should enable students to:
- Graph conic sections.
- Solve problems involving their equations.
9. Trigonometry in Algebra 2 Worksheet

Trigonometry extends the application of algebra:
- Sine, cosine, and tangent functions.
- Angles in standard position.
- Trigonometric identities and proofs.
Worksheet content should include:
- Verification of trigonometric identities.
- Graphing trigonometric functions.
- Solving trigonometric equations.
10. Probability and Statistics Worksheet

Statistics and probability topics often included in Algebra 2:
- Measures of central tendency and spread.
- Basic probability rules.
- Normal distribution.
Students should practice:
- Calculating probabilities.
- Using statistical charts and graphs.
- Applying binomial theorem for probability problems.
These worksheets collectively address a broad range of topics critical for Algebra 2 mastery. They provide ample opportunities for students to practice and gain proficiency in solving complex problems. As students work through these worksheets, they should:
- Recognize the importance of understanding basic algebraic concepts before tackling advanced topics.
- Learn different problem-solving strategies and when to apply them.
- Develop fluency in using algebraic methods to solve real-life scenarios.
In summary, Algebra 2 extends the boundaries of basic algebra, introducing new and more complex problems that require deeper understanding and skill. These 10 worksheets serve as essential tools for students to practice, reinforce, and apply their algebraic knowledge, fostering an environment conducive to deep learning and mathematical mastery.
Why is it important to master Algebra 2?

+
Algebra 2 provides the foundation for higher-level mathematics such as Calculus, Trigonometry, and beyond. It introduces students to abstract thinking, problem-solving techniques, and the complexity of mathematical systems, which are crucial for careers in STEM fields.
How can these worksheets be integrated into a classroom setting?

+
Teachers can use these worksheets for in-class practice, homework assignments, or as part of a testing regime. They can be combined with interactive lessons, group work, or individual study sessions to reinforce learning and provide varied learning experiences.
What should students do if they are struggling with a particular worksheet?

+
Students should review the foundational concepts of Algebra 2, seek additional resources or tutoring, work in study groups, and ask their teacher for guidance. Breaking down complex problems into smaller, manageable parts can also help in understanding the underlying math.