Algebra 1 Slope Intercept Form: Worksheet Mastery

Understanding the slope-intercept form of a line is a fundamental concept in Algebra 1 that helps students predict the behavior of linear equations. By mastering this form, students can visualize lines on a graph, understand how changes in the equation affect the line, and solve real-world problems involving linear relationships. This blog post will guide you through a comprehensive worksheet designed to enhance your understanding of the slope-intercept form, y = mx + b.
What is Slope-Intercept Form?

Before we dive into the worksheet, let’s quickly review what the slope-intercept form represents:
- m: The slope of the line, which tells us how steep the line is or how much the y-value changes for each unit increase in x.
- b: The y-intercept, which is the point where the line crosses the y-axis, telling us the starting value of y when x is 0.
With this form, we can instantly identify two critical pieces of information about a line:
- How quickly the line rises or falls
- Where it starts on the y-axis

Worksheet Design

The worksheet is divided into several sections to ensure that students gain a thorough understanding:
Section 1: Identifying Slope and Y-Intercept
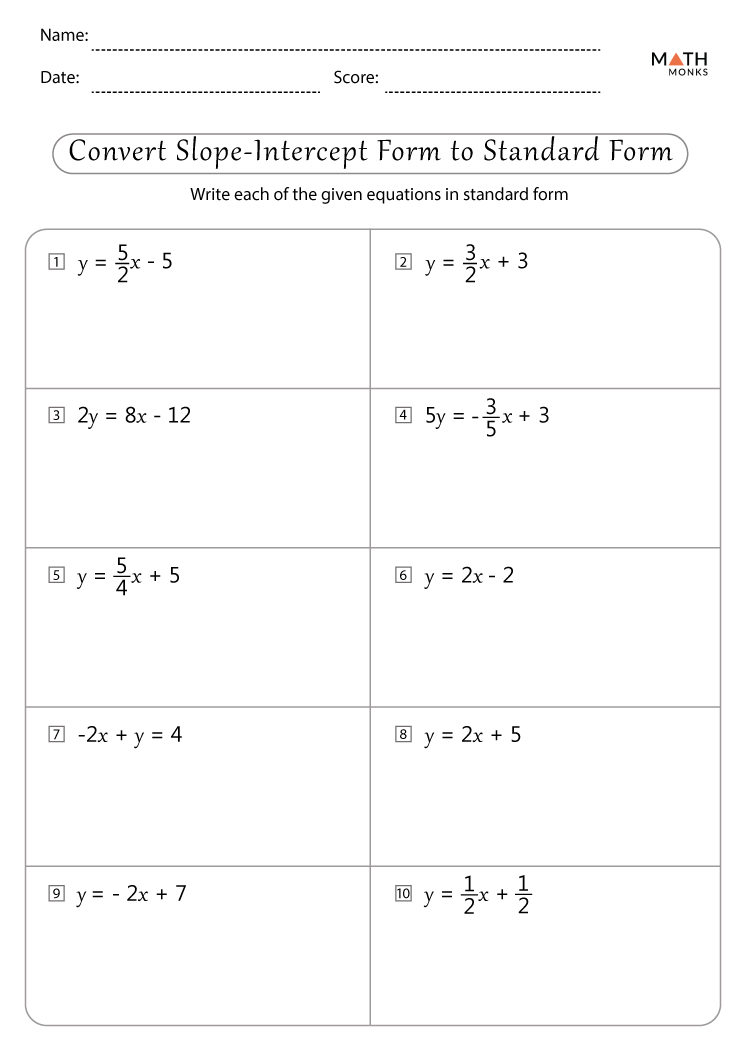
This part of the worksheet focuses on extracting m and b from given linear equations. Here, students are presented with equations like:
| Equation | Slope (m) | Y-Intercept (b) |
|---|---|---|
| y = 2x + 5 | 2 | 5 |
| y = -0.5x + 7 | -0.5 | 7 |
| y = 1 | 0 | 1 |

💡 Note: Not all equations are in standard slope-intercept form. Students must learn to manipulate equations to see them in this form.
Section 2: Graphing Lines from Slope-Intercept Form

Students are tasked with plotting lines on a coordinate plane given their slope and y-intercept. This activity helps solidify:
- How to place the y-intercept on the graph
- How to draw a line through this point at the given slope
Examples include:
- Plot the line with a slope of 2 and a y-intercept of -3.
- Sketch the line with a slope of -1/3 and a y-intercept of 4.
Section 3: Converting Between Different Forms

Here, students convert equations from standard form or point-slope form to slope-intercept form, and vice versa. This practice is crucial for:
- Understanding equivalence between different equation forms
- Practical application in real-life problems
Section 4: Word Problems

This section presents real-world scenarios where students must:
- Determine the slope based on rate of change
- Identify the y-intercept as an initial value
- Formulate and solve equations
Examples might include:
- A student's allowance increases by $2 per week, starting at $10. How can this be expressed in slope-intercept form?
Section 5: Advanced Slope-Intercept Problems

These problems challenge students with:
- Slope calculation through a pair of points
- Determining equations from parallel or perpendicular lines
Key Takeaways

Through this worksheet, students will:
- Gain proficiency in identifying slopes and y-intercepts from equations
- Develop skills in graphing linear equations
- Understand the relationship between different forms of linear equations
- Apply their knowledge to practical problems
🔍 Note: The versatility of slope-intercept form extends beyond mathematics to various fields like physics, economics, and engineering.
By working through these exercises, students will enhance their algebra skills, preparing them for more complex mathematical concepts in higher education and professional settings.
How do you find the slope-intercept form of an equation?

+
To find the slope-intercept form of an equation, you need to ensure the equation is written in the form y = mx + b, where m is the slope and b is the y-intercept.
Why is the y-intercept important?

+
The y-intercept indicates the point where the line crosses the y-axis, which often represents the initial value in real-world scenarios.
Can slope-intercept form be used for vertical lines?

+
No, vertical lines cannot be represented in slope-intercept form since they have an undefined slope.
How does changing the slope affect a line’s graph?

+
Increasing the slope makes the line steeper, while decreasing it makes the line flatter.
What real-life scenarios can be modeled by slope-intercept form?
+
Applications include cost analysis (where slope represents cost per unit), depreciation, physics (velocity-time graphs), and economics (growth rates).



