Algebra 1 Multi Step Equations Practice Worksheet
Mastering algebra is not just about understanding basic operations but also about solving complex problems efficiently. Algebra 1 multi-step equations are a critical part of this journey. In this blog post, we will dive deep into the concept of multi-step equations, provide practical examples, and guide you through a detailed practice worksheet to enhance your problem-solving skills.
Understanding Multi-Step Equations

Before we delve into the complexities, it's essential to understand what multi-step equations are:
- They require more than one arithmetic operation to solve.
- Often involve distributing, combining like terms, and isolating the variable.
- Practical in real-world scenarios where you need to find a variable value amidst multiple conditions.
🔍 Note: Multi-step equations build on basic algebra concepts, making algebra more versatile and applicable to various problem-solving contexts.
Key Steps in Solving Multi-Step Equations

- Distribute: If there are parentheses, distribute any constants or variables outside them.
- Combine Like Terms: Simplify by adding or subtracting like terms on both sides of the equation.
- Isolate the Variable: Use inverse operations to get the variable alone on one side of the equation.
- Check Your Work: Always verify your solution by substituting it back into the original equation.
✍️ Note: Each step should be clearly understood as it sets the foundation for more complex algebra operations.
Example Walkthrough

Let's consider a simple equation to explain the process:
Example Equation: 3(x + 5) = 18
Step-by-Step Solution:

- Distribute: 3x + 15 = 18
- Subtract 15: 3x = 3 (to isolate 3x, we subtract 15 from both sides)
- Divide by 3: x = 1 (now we divide both sides by 3 to get x by itself)
🔍 Note: When isolating the variable, ensure that you perform the same operation on both sides of the equation to keep it balanced.
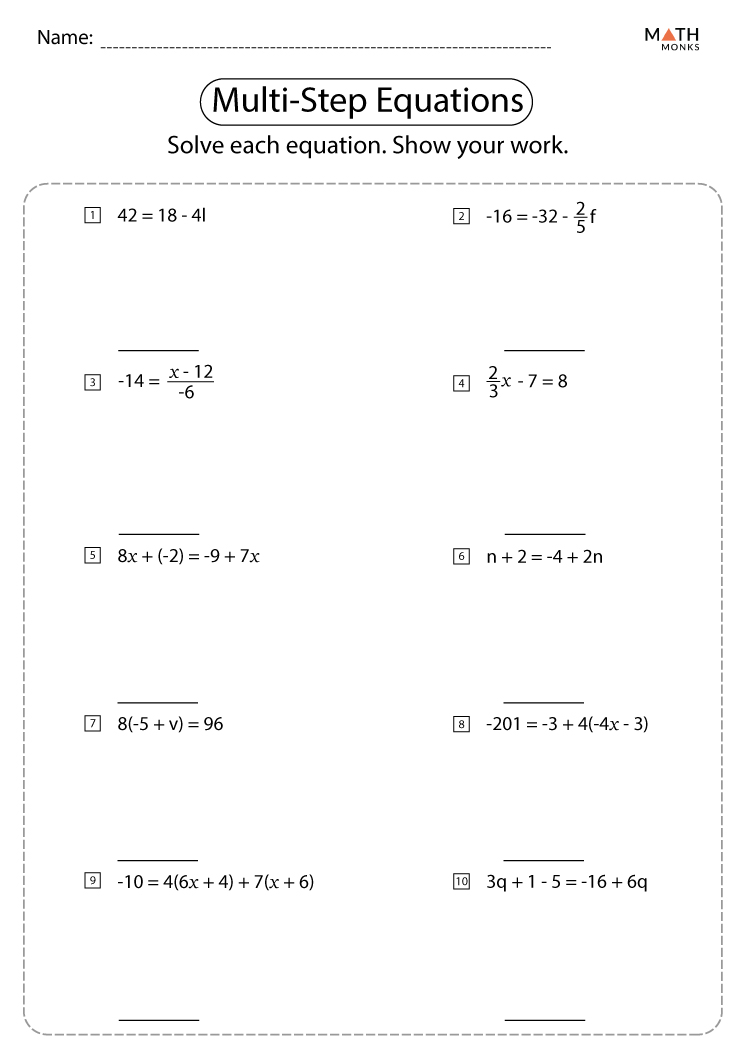
Multi-Step Equations Practice Worksheet

Now let's enhance your algebra skills with some practice. Here's a worksheet designed to challenge and improve your understanding:
| Problem | Solution Steps |
|---|---|
| 1. 2x + 3 = 15 |
|
| 2. 5(y - 4) = 25 |
|
| 3. 4a + 6 - a = 27 |
|
| 4. -2(x + 3) + 5 = 1 |
|
| 5. (1/2)c - 3 = -5 |
|
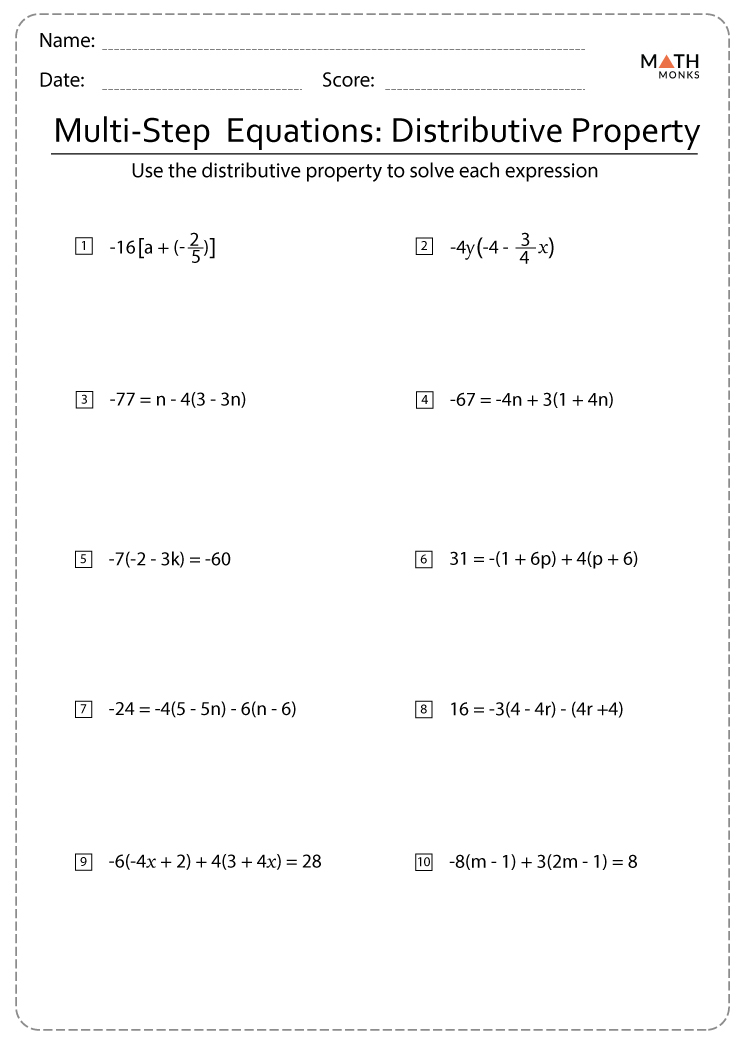
📚 Note: Regular practice with a variety of problems ensures a well-rounded understanding of algebraic principles.
Additional Tips for Solving Multi-Step Equations
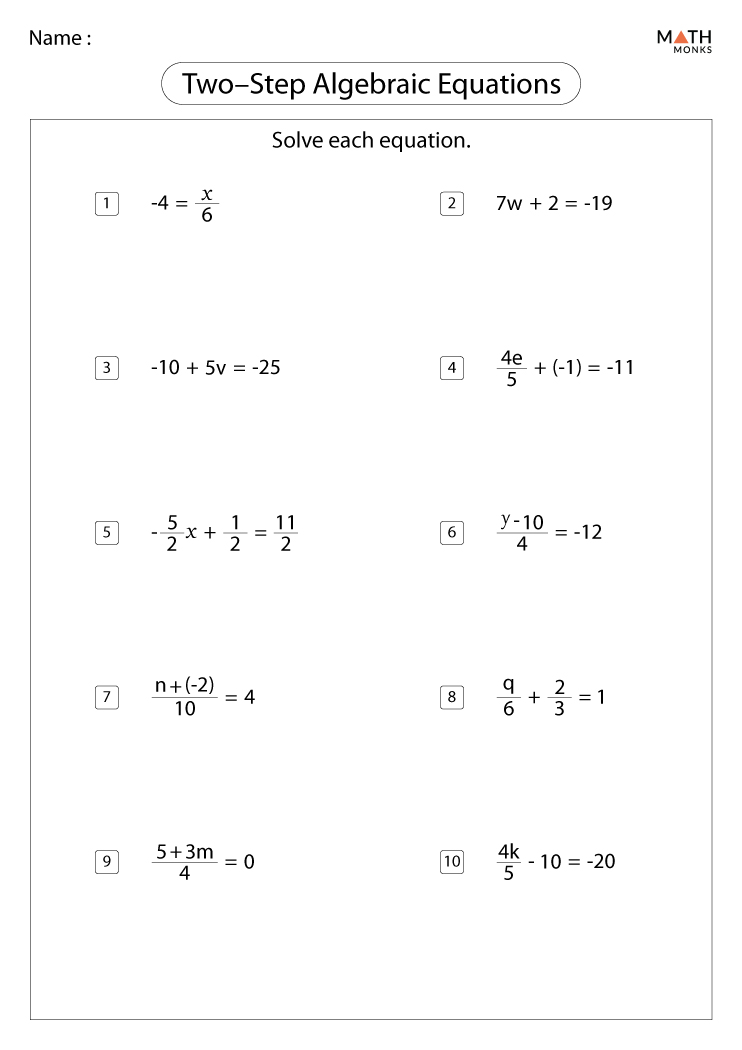
- Understand Order of Operations: Always follow PEMDAS (Parentheses, Exponents, Multiplication and Division, Addition and Subtraction).
- Use Graph Paper: Writing equations on graph paper can help maintain organization.
- Double Check: After solving, substitute your answer back to check for consistency.
🎓 Note: Mastery comes with time and consistent practice. Don't rush the process; enjoy learning and overcoming each challenge.
In this detailed exploration, we've covered the essentials of multi-step equations, from foundational concepts to practical examples and exercises. Understanding and solving these equations not only hones your algebra skills but also prepares you for more complex mathematical concepts. Remember, the key to success in algebra is consistent practice and a logical approach to problem-solving. Each step in algebra is a step toward a broader understanding of mathematics and its application in various real-world scenarios.
Why are multi-step equations important?

+
Multi-step equations are crucial because they reflect real-life problems where multiple variables and steps are involved. They teach structured problem-solving and reinforce foundational algebraic concepts.
How can I improve in solving multi-step equations?

+
Regular practice with different types of equations, understanding the logic behind each step, and consistent application of algebraic rules will help improve your skills. Visual aids and working with peers can also be beneficial.
What are common mistakes in solving multi-step equations?

+
Frequent errors include forgetting to distribute a negative number, miscombining terms, or performing incorrect operations (like dividing by zero). Ensuring each step is correctly executed can prevent these mistakes.
Can multi-step equations be applied in real life?

+
Absolutely. From calculating taxes to determining profits or even solving physics problems, multi-step equations are often at the core of many practical scenarios.
Are there resources available for further practice?

+
Yes, numerous educational websites offer free worksheets, tutorial videos, and practice problems. Libraries and educational forums also provide resources tailored to algebra practice.