5 Simple Tips for Polynomial Operations Worksheet Success
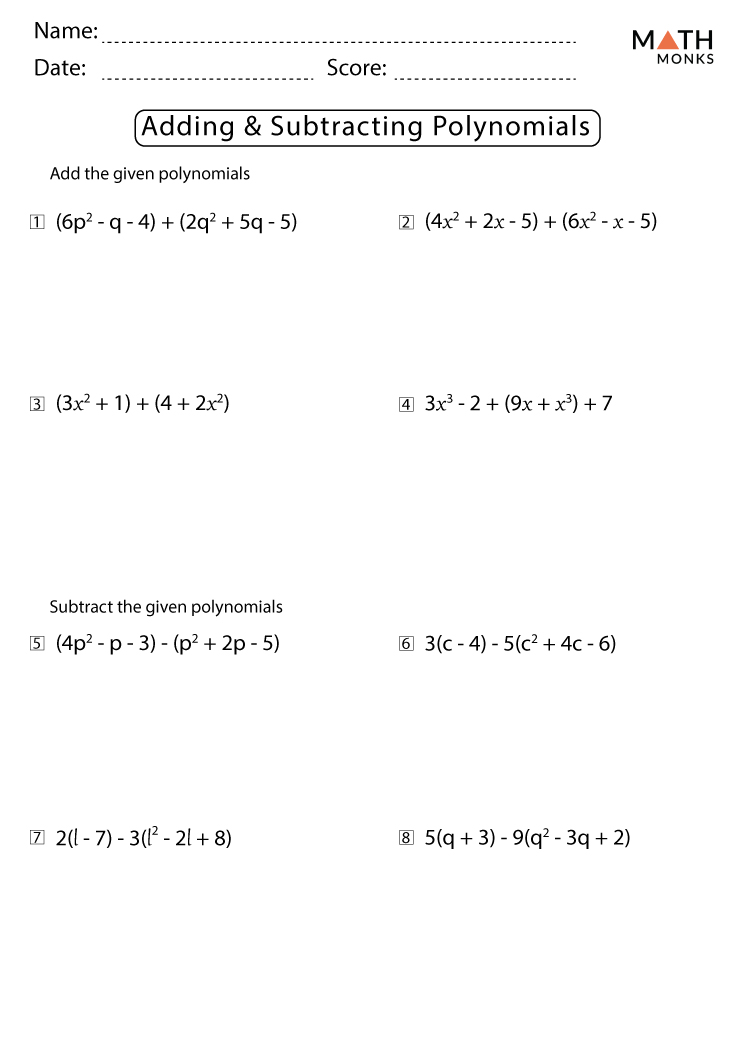
Polynomial operations can often be one of the more challenging aspects of algebra for students. Whether you're dealing with addition, subtraction, multiplication, or division of polynomials, having a structured approach can make all the difference. Here are five simple tips to help you navigate polynomial operations successfully.
Tip 1: Understand the Basics

Before diving into the complexities of polynomial operations, ensure you have a solid understanding of the basics:
- Polynomial Definition: A polynomial is an expression consisting of variables and coefficients, involving only the operations of addition, subtraction, multiplication, and non-negative integer exponents of variables.
- Like Terms: When working with polynomials, knowing how to identify like terms is crucial. These are terms with the same variable raised to the same power.
- Coefficients and Exponents: Understand how these elements behave in operations.
Tip 2: Use the Box Method for Multiplication
The box method or area method can be particularly helpful when multiplying two polynomials:
- Draw a grid or box with as many rows and columns as there are terms in each polynomial.
- Label the sides with the terms of each polynomial.
- Multiply each pair of terms where the row meets the column and write the result inside the box.
- Combine like terms to simplify the expression.

Tip 3: Employ the Distributive Property

When dealing with polynomial multiplication or factoring, the distributive property is your best friend:
- Distribute each term inside the parenthesis to each term outside, and then combine like terms.
For example, if you need to multiply (2x + 3) by (x + 5):
| Polynomials | Operation | Result |
|---|---|---|
| (2x + 3) * (x + 5) | Distribution | 2x * x + 2x * 5 + 3 * x + 3 * 5 |
| After distribution | 2x^2 + 10x + 3x + 15 | |
| Combine like terms | 2x^2 + 13x + 15 |

Tip 4: Factorize for Easier Division

Dividing polynomials can be made simpler by factoring:
- Factorize the numerator and the denominator.
- Look for common factors to cancel out.
- Simplify what remains.
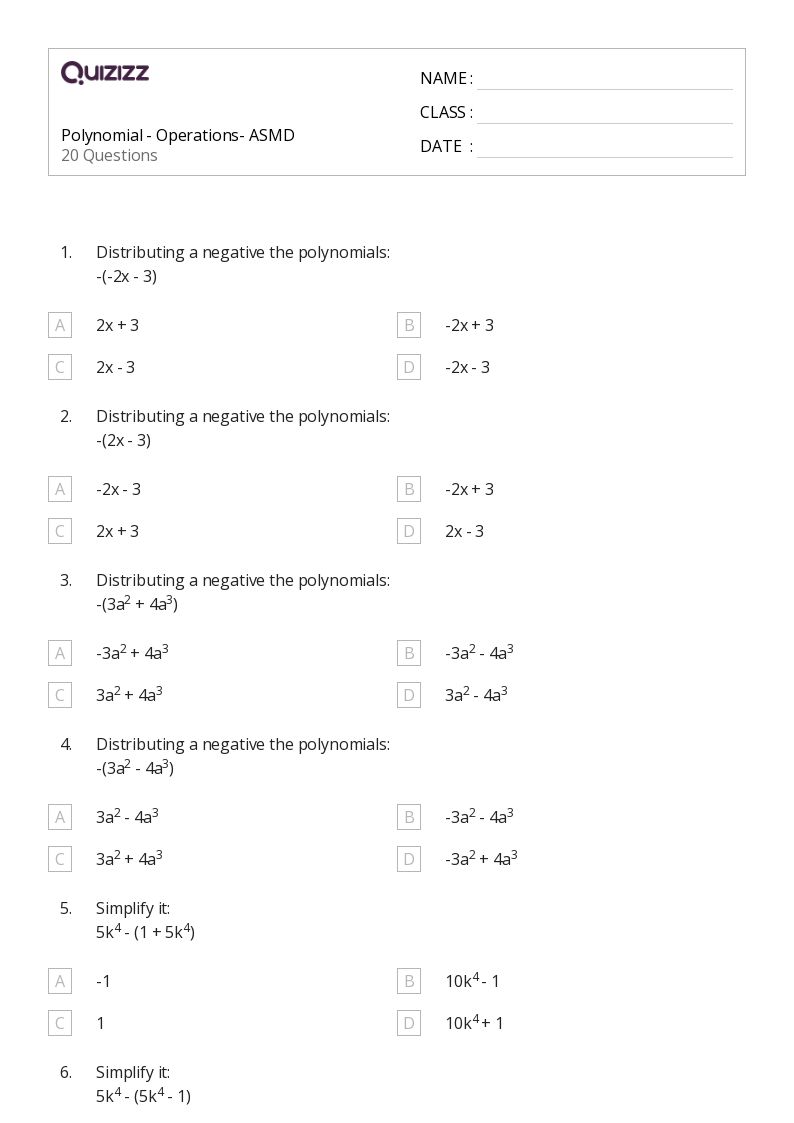
Tip 5: Practice with a Systematic Approach

Success in polynomial operations often comes down to practice:
- Start with simple problems and gradually increase complexity.
- Use polynomial operations worksheets to reinforce your understanding.
- Keep track of your common mistakes and learn from them.
✅ Note: Regular practice is key to mastering polynomial operations. Don't just rely on theory; apply it in your worksheets and exercises.
By integrating these five tips into your study routine, you'll find polynomial operations becoming much more manageable. Understanding the basics, employing the box method for multiplication, using the distributive property, factoring for division, and practicing systematically will not only enhance your algebra skills but also boost your confidence in tackling polynomial problems. As you continue to work through your polynomials, remember that consistent effort and a clear understanding of foundational concepts are your best tools for success.
Why do we need to understand like terms?

+
Like terms are crucial in polynomial operations because they allow us to simplify expressions. When terms have the same variable raised to the same power, they can be combined, which reduces complexity and helps in solving or simplifying the polynomial.
What are the benefits of using the box method in polynomial multiplication?

+
The box method visually organizes the multiplication of polynomials. It breaks down the multiplication into simpler steps, making it less error-prone and easier to understand, especially for beginners.
How does the distributive property help in polynomial operations?

+
The distributive property allows you to distribute multiplication across addition or subtraction within polynomials. This not only simplifies the multiplication process but also helps in factoring and solving polynomial equations.
Can you always factor polynomials to simplify division?

+
No, not all polynomials can be factored easily. However, when possible, factoring can significantly simplify division by allowing you to cancel out common factors. For polynomials that cannot be factored, long division or synthetic division methods might be necessary.
What is the best way to practice polynomial operations?

+
The best practice involves using structured worksheets, increasing complexity gradually, analyzing your mistakes, and understanding the underlying concepts. Regular, focused practice sessions, coupled with reviewing theory, will lead to proficiency in polynomial operations.