Mastering Fractions: Adding Different Denominators Worksheet

In the realm of arithmetic, the task of adding fractions with different denominators can often seem like a daunting challenge. However, with the right approach, this becomes a skill easily within anyone's grasp. This comprehensive guide will walk you through the process of mastering the addition of fractions with different denominators, a key component of mathematics for students at various educational levels.
Understanding Fractions

What is a Fraction? A fraction represents a part of a whole or a collection. It consists of a numerator (the top number) which tells us how many parts we are considering, and a denominator (the bottom number) which indicates how many parts the whole has been divided into.

Adding Fractions: The Basics

Before diving into the complexities of different denominators, here's a brief reminder on how to add fractions with the same denominator:
- Keep the denominator the same.
- Add the numerators together.
- If possible, simplify the fraction.
Why Do Different Denominators Matter?

When the denominators of two fractions differ, you can't directly add them. Here’s why:
- Denominators represent different sizes of the whole.
- Direct addition would lead to an incorrect total because you're essentially adding different sized pieces together.
The Two Main Approaches to Add Fractions with Different Denominators

Method 1: Finding a Common Denominator
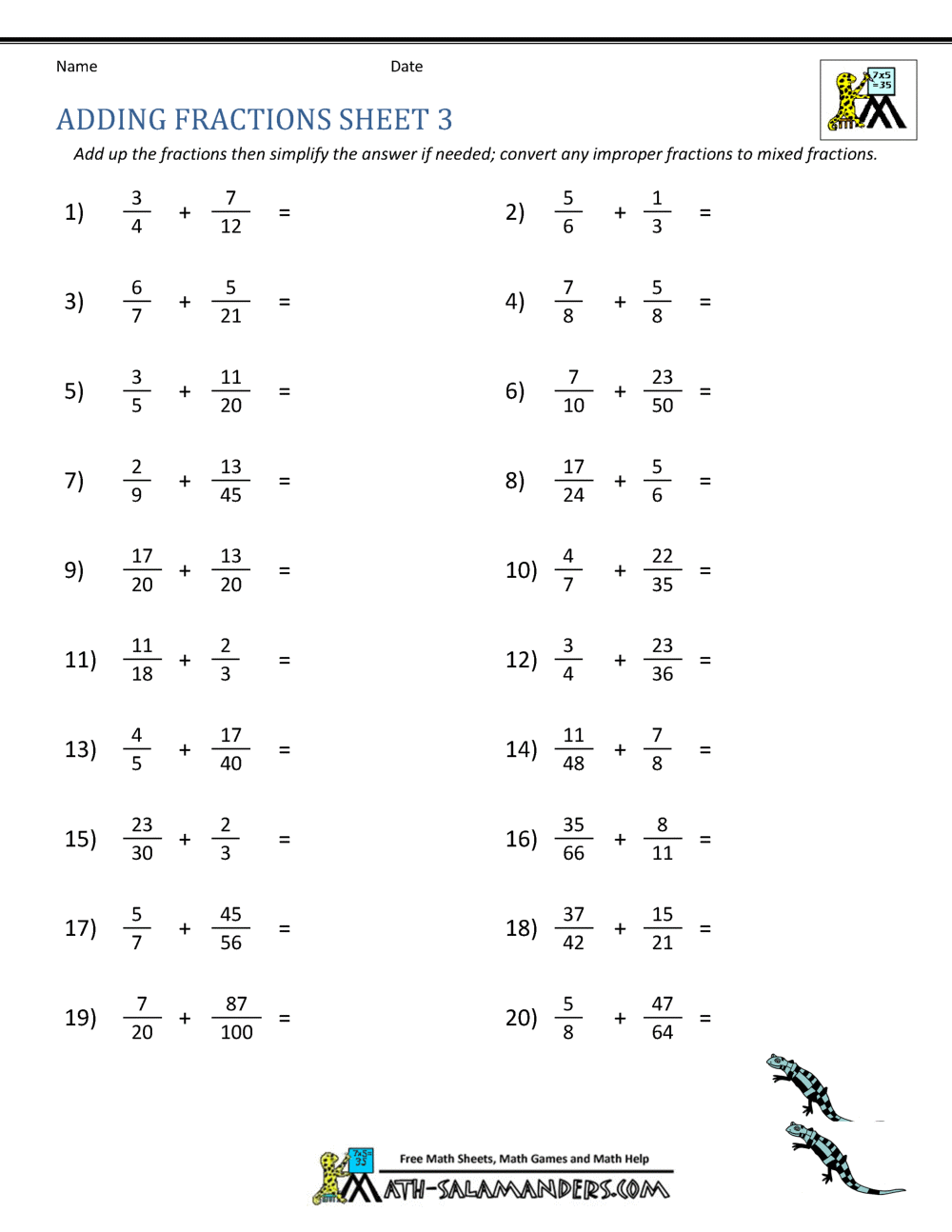
To add fractions with different denominators, one needs to find a common denominator, which is the least common multiple (LCM) of the denominators:
- Determine the LCM: Find the LCM of both denominators. This is often easier if you list the multiples of each denominator.
- Convert each fraction: Multiply both the numerator and the denominator by the appropriate factor to make each denominator equal to the LCM.
- Add the numerators: With the denominators now the same, add the numerators.
- Simplify if possible: Reduce the resulting fraction to its simplest form.
Example:
| Step | Fraction 1 | Fraction 2 |
| Original | 1⁄3 | 1⁄4 |
| LCM | 12 (3 x 4 = 12) | |
| Converted | 4⁄12 | 3⁄12 |
| Added | 7⁄12 | |

💡 Note: Finding the LCM manually can be time-consuming, but it is beneficial in understanding the process deeply.
Method 2: Using the Butterfly Method

The Butterfly Method, also known as the cross-multiplication method, provides an alternative visual approach for adding fractions with different denominators:
- Cross-multiply: Multiply the numerator of one fraction by the denominator of the other to get two new numbers.
- Add the two products: Sum the results from step 1.
- Add the denominators: Add the denominators together.
- Form the new fraction: Place the sum of the products over the sum of the denominators.
- Simplify: If the resulting fraction can be simplified, do so.
Example:
- 1⁄3 + 1⁄4 = (1x4 + 1x3) / (3 + 4) = 7⁄7
💡 Note: The Butterfly Method is quicker for small numbers but can become cumbersome with larger values.
Practical Tips for Adding Fractions

- Ensure common denominators: This is fundamental to correct addition.
- Practice: The more you practice, the faster you’ll get at finding common denominators or using the Butterfly Method.
- Remember the rules: Simplify your result, if necessary, to keep your fraction in the simplest form.
To summarize, adding fractions with different denominators is not as hard as it might initially appear. Whether through finding a common denominator or using the Butterfly Method, the key is understanding the relationship between fractions. By converting all fractions to have a common denominator, you are essentially speaking the same "language," allowing for easy addition. The Butterfly Method offers a quick visual approach, though it might not be as intuitive for everyone. Practical application and practice will turn these techniques into second nature, enhancing your mathematical prowess in handling fractions.
What if the fractions have mixed numbers?

+
Convert mixed numbers to improper fractions first, then follow the steps to add fractions with different denominators.
Is it necessary to always simplify my results?

+
While not always necessary for the accuracy of the calculation, simplifying helps in presenting the fraction in its simplest form, making it easier to understand and compare.
Can I use these methods for subtraction as well?

+
Yes, the same process applies for subtraction of fractions with different denominators. Instead of adding the numerators, you would subtract them.