Adding Exponents With Same Base Worksheet

Mathematics can seem daunting to many students, yet understanding certain core concepts, such as exponents, can unlock a myriad of problem-solving skills. When working with exponents, one of the foundational operations students learn is how to add exponents with the same base. This process not only simplifies mathematical expressions but also helps in grasping how numbers grow or shrink exponentially, a principle that has practical applications in various fields like finance, computing, and science. This post will provide a detailed worksheet for adding exponents with the same base, ensuring you understand the concept thoroughly.
Why Add Exponents?

Exponents, or powers, indicate the number of times a base number is multiplied by itself. When you’re working with terms that have the same base, adding exponents can significantly streamline algebraic expressions. Here are a few reasons why understanding this operation is essential:
- Simplification: Combining like terms with exponents reduces the complexity of expressions, making them easier to solve or interpret.
- Real-world Applications: From calculating compound interest to understanding scientific notation, exponent addition is used in diverse real-life scenarios.
- Mathematics Building Block: It’s a stepping stone for more complex algebraic and calculus concepts where exponents frequently appear.
Basic Rules for Adding Exponents

Before we dive into the worksheet, let’s recap the basic rules for adding exponents:
- Same Base: You can only add exponents if the bases are the same.
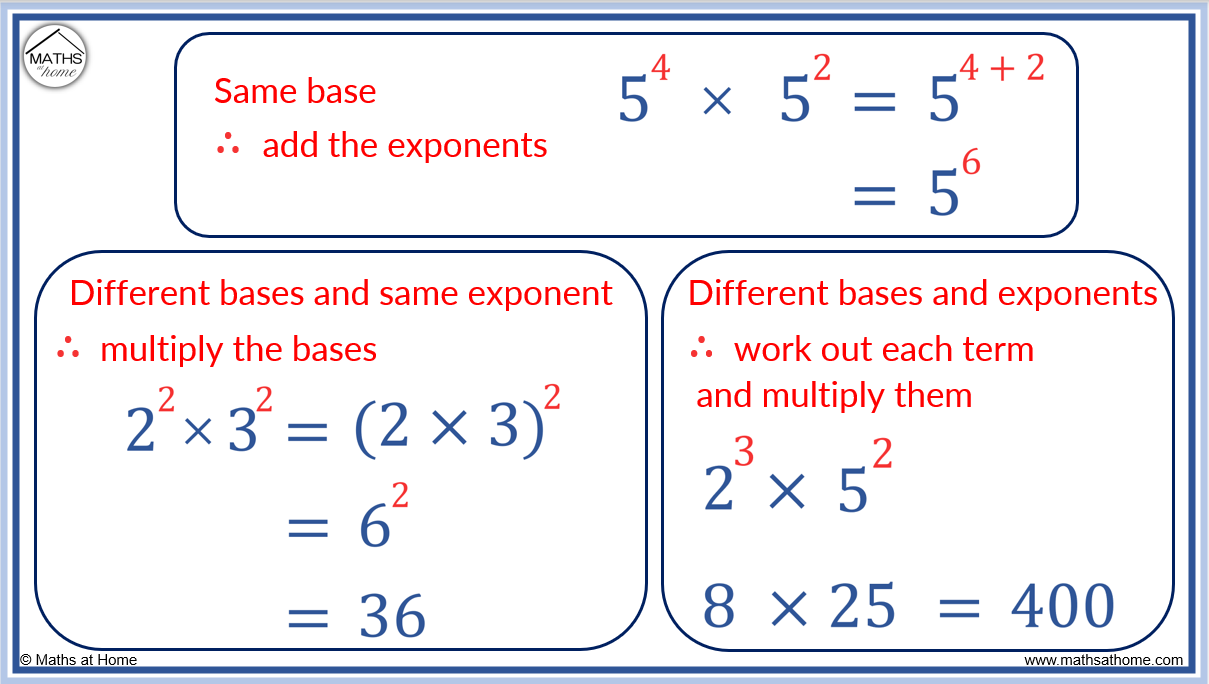
- Exponent Rule: If the bases are the same, you add their exponents. This rule is symbolized as: [ a^m + a^n = a^{m+n} ]
- Example: (2^2 + 2^3 = 2^{2+3} = 2^5)
Adding Exponents with Same Base Worksheet

Instructions:
Below is a worksheet designed to give you practical experience with adding exponents. Solve each problem, and remember to show your work. Here are the problems:
| Problem | Solution |
|---|---|
| 1. 3^4 + 3^2 = ? | \[3^4 + 3^2 = 3^{4+2} = 3^6 = 729\] |
| 2. 5^3 + 5^1 = ? | \[5^3 + 5^1 = 5^{3+1} = 5^4 = 625\] |
| 3. x^5 + x = ? | \[x^5 + x = x^{5+1} = x^6\] |
| 4. 2a^2 + a^4 = ? | \[2a^2 + a^4 = a^2(2 + a^2)\] |
| 5. z^4 + z^2 + z = ? | \[z^4 + z^2 + z = z^{4} + z^{2} + z^{1} = z^{4+2+1} = z^7\] |

Notes:

💡 Note: These are basic problems to help you understand the concept. Real-world problems might require more steps, like factoring out common factors before adding exponents.
In this worksheet, we've covered the simplest form of adding exponents with the same base. Here are some key takeaways:
- When adding exponents with the same base, you simply add the powers together.
- Remember that you can only add exponents if the base is the same.
- The process helps in simplifying complex mathematical expressions, making them easier to work with.
Understanding the addition of exponents with the same base is not just an academic exercise but a fundamental skill that aids in grasping higher levels of algebra. It lays the groundwork for manipulating equations, understanding growth rates in finance, and decoding scientific notations, to name a few practical applications.
What happens if the bases are not the same?

+
If the bases are different, you cannot add the exponents directly. Instead, you’d need to find a common base or simplify the terms individually.
Can I apply this rule to all types of exponents?

+
Yes, the rule applies to integer, rational, and even complex exponents as long as the bases are identical.
What are some real-world examples of adding exponents with the same base?

+
Examples include calculating compound interest where the number of times interest is compounded acts as the exponent, or in scientific notation when combining values like (10^3 + 10^5).
Is there a graphical representation of this rule?

+
Yes, if you graph functions with exponents like (y = 3^x) and (y = 3^{x+2}), the latter function would simply be a horizontal shift of the former by 2 units to the left.
Can adding exponents ever give you a negative result?

+
When adding exponents with the same base, the result won’t be negative as long as you’re dealing with positive bases and exponents. However, if you have negative bases or exponents, you must consider the sign rules for exponents.