5 Essential Tips for Unlike Denominator Fraction Worksheets

When tackling the subject of unlike denominators in fractions, it's common for students to face a mix of confusion and frustration. Yet, mastering this can be significantly rewarding and enhances mathematical proficiency. This blog post dives deep into 5 Essential Tips for Unlike Denominator Fraction Worksheets, offering educators, parents, and students practical strategies to conquer this topic. Let's explore how to simplify this complex mathematical area in an engaging and understandable manner.
The Importance of Understanding Unlike Denominator Fractions


Fractions with unlike denominators are essential in mathematics because they are encountered in everyday problem-solving and advanced algebra. Understanding how to manipulate these fractions:
- Provides a solid foundation for arithmetic, algebra, and calculus.
- Is crucial for practical applications like measurements, recipes, and financial planning.
Tip 1: Start with a Common Foundation

Before diving into the complexity of unlike denominators:
- Teach and review the concept of equivalent fractions. Ensure students understand that fractions can represent the same value even if the numerator and denominator are different.
- Utilize visual aids like fraction strips or pie charts to demonstrate how fractions relate to each other.
🔍 Note: Using visual aids not only helps in understanding but also keeps students engaged.
Tip 2: Emphasize the Least Common Denominator (LCD)


The concept of the least common denominator is pivotal when dealing with unlike denominators. Here’s how to teach it:
- Introduce prime factorization as a method to find the LCD. Explain how to determine the smallest number that both denominators can divide into evenly.
- Illustrate with examples how to convert fractions to have the same denominator using the LCD.
| Denominators | Prime Factors | LCD |
|---|---|---|
| 6, 8 | 6 = 2 × 3, 8 = 2 × 2 × 2 | 24 |

⚠️ Note: Emphasizing prime factorization reduces the chances of errors when finding the LCD.
Tip 3: Practice with Conversion
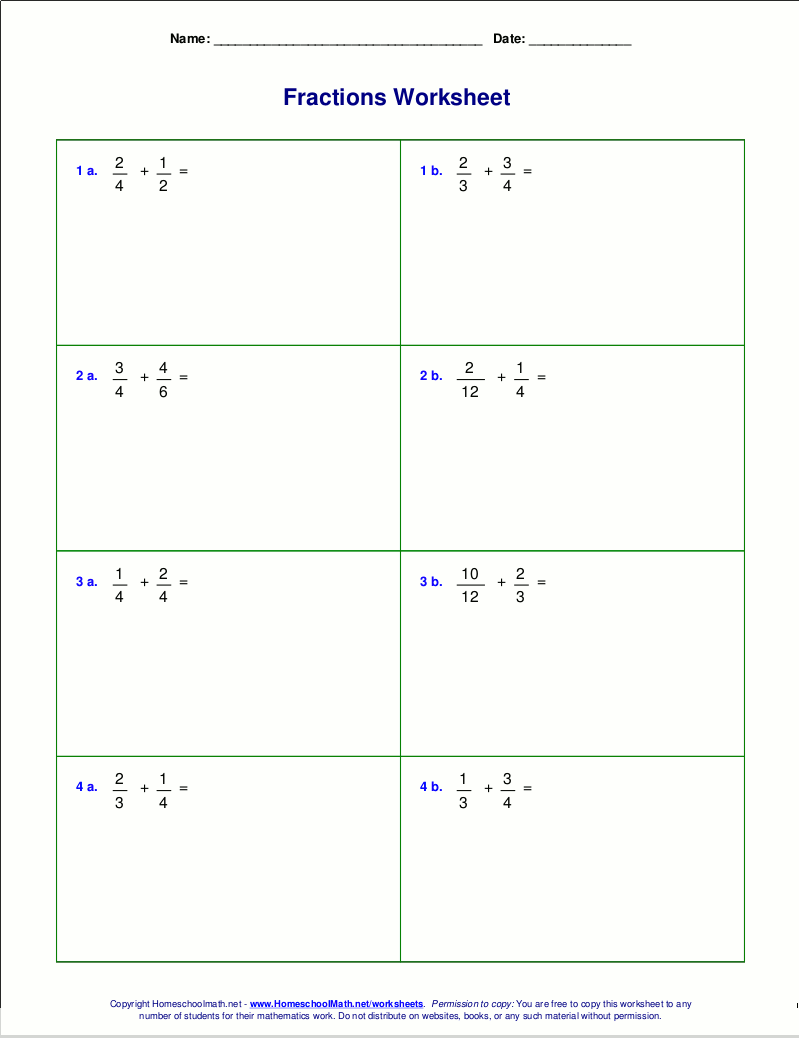
Practicing the conversion of fractions to have a common denominator is key:
- Create exercises where students must convert multiple fractions to a common denominator. Encourage them to think through each step logically.
- Use real-life scenarios or interactive games to make the practice session fun and educational.
Tip 4: Master Addition and Subtraction


When it comes to adding or subtracting fractions with unlike denominators:
- Teach the steps sequentially: finding the LCD, converting, then performing the operation, and simplifying the result if necessary.
- Use mnemonics or simple rhymes to help students remember the sequence of operations.
👁️ Note: Mnemonics can significantly aid in memorizing procedural steps for complex operations.
Tip 5: Use Real-Life Applications

Relate unlike denominator fractions to real-life situations to make the learning experience relevant:
- Discuss examples like splitting a pizza among different groups, calculating time for overlapping events, or even measuring ingredients for a recipe.
- Encourage students to bring in or think of their own examples where they’ve encountered fractions in daily life.
As we’ve journeyed through these essential tips, it’s clear that understanding unlike denominator fractions is not just about academic success but also about making sense of the world around us. By grounding the abstract concepts in tangible applications, practicing methodically, and using visual aids, students can build confidence in their mathematical skills. This approach not only demystifies unlike denominator fractions but also instills a love for learning and problem-solving.
Why is finding the LCD important?

+
The LCD ensures that fractions have the same denominator, making addition and subtraction possible without changing the value of the fractions.
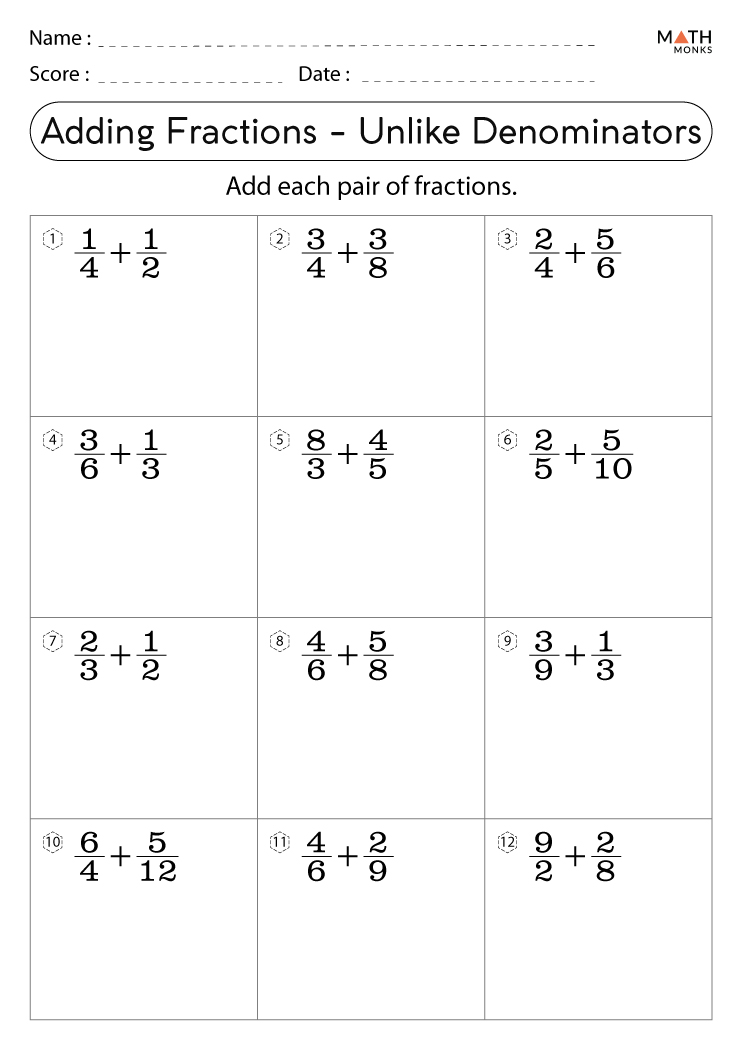
How can visual aids help with unlike denominators?
+
Visual aids provide a concrete representation of abstract fractions, allowing students to visualize how fractions relate to each other.
Can unlike denominator fractions be used in real-life scenarios?

+
Yes, unlike denominator fractions appear in various real-life contexts like baking, budgeting, and measuring time or distances.