5 Essential Worksheets for Mastering Exponent Operations
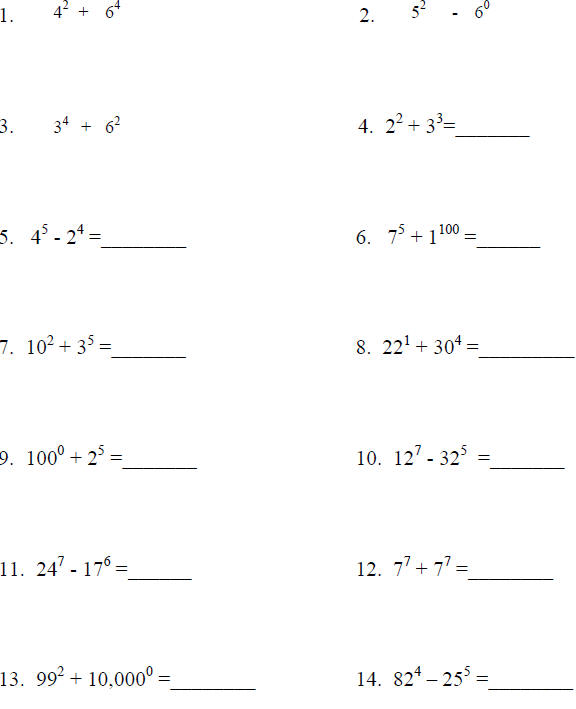
Exponent operations are fundamental in various fields of mathematics, from basic arithmetic to advanced calculus and beyond. They enable us to handle multiplication and division in a more efficient and compact manner. For students and mathematicians alike, mastering exponents is not just about understanding the rules; it's about applying them in various contexts. In this comprehensive guide, we'll delve into five essential worksheets that are tailored to help anyone from beginners to advanced learners in grasping the nuances of exponent operations.
Worksheet 1: Basic Exponent Rules

Understanding basic exponent rules forms the foundation for all further operations. This worksheet is designed for students who need to reinforce their understanding:
- Product of Powers Rule: ( a^m \times a^n = a^{m+n} )
- Quotient of Powers Rule: ( \frac{a^m}{a^n} = a^{m-n} )
- Power to a Power Rule: ( (a^m)^n = a^{mn} )
- Product to a Power Rule: ( (ab)^n = a^n \times b^n )
- Zero Exponent Rule: ( a^0 = 1 )
💡 Note: Ensure students practice with both positive and negative bases and exponents to cover all scenarios.
Worksheet 2: Simplifying Expressions

Simplifying expressions involving exponents can be daunting, but with practice, it becomes second nature. This worksheet includes problems like:
- ( (3^2 \times 3^4) \div 3^3 )
- ( \frac{7^5 \times 7^{-3}}{7^4} )
| Problem | Example |
|---|---|
| Simplify ( (3^2 \times 3^4) \div 3^3 ) | ( 3^{2+4-3} = 3^3 ) |
| Simplify ( \frac{7^5 \times 7^{-3}}{7^4} ) | ( 7^{5 + (-3) - 4} = 7^{-2} ) |

Worksheet 3: Negative Exponents

Dealing with negative exponents can be confusing initially, but this worksheet helps clarify:
- ( 2^{-3} = \frac{1}{2^3} = \frac{1}{8} )
- ( 5^{-2} = \frac{1}{5^2} = \frac{1}{25} )
🔍 Note: Remind students that moving a number from the numerator to the denominator or vice versa changes the sign of its exponent.
Worksheet 4: Scientific Notation and Exponents

Scientific notation uses exponents to write large or small numbers efficiently. This worksheet includes:
- Convert 0.000006 into scientific notation: ( 6 \times 10^{-6} )
- Convert 3,500,000 into scientific notation: ( 3.5 \times 10^6 )
Worksheet 5: Logarithms and Exponents
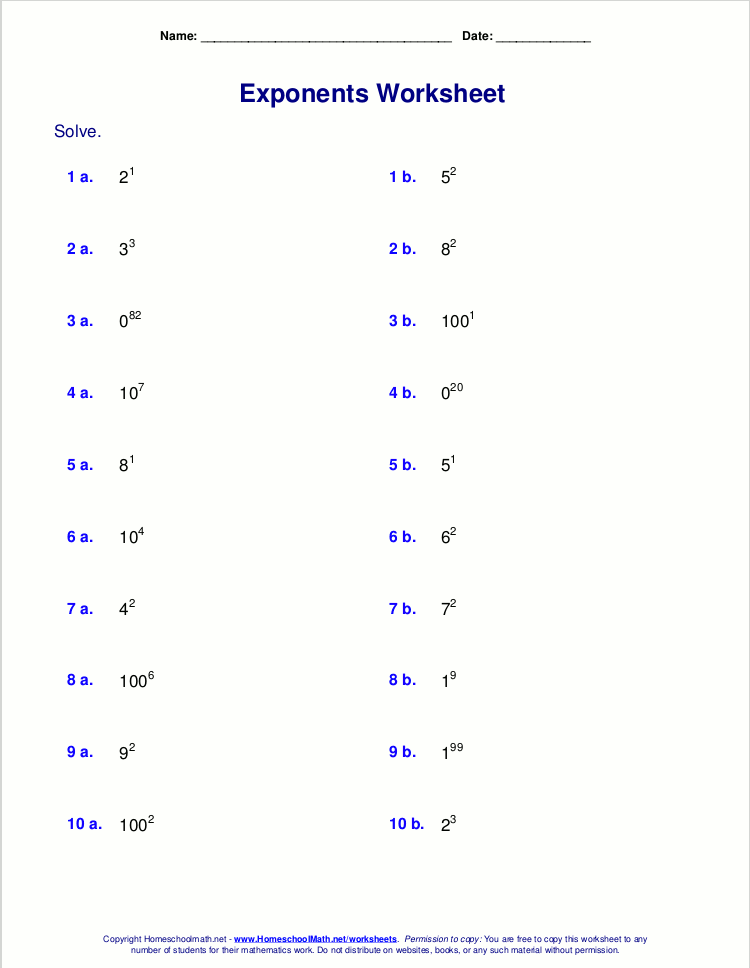
Logarithms are closely related to exponents, providing another perspective on operations with exponents:
- Evaluate ( \log_2(8) )
- Evaluate ( \log_3(27) )
📈 Note: Understanding logarithms and exponents together can significantly enhance problem-solving skills in algebra and calculus.
Throughout these worksheets, the focus is not only on performing operations but also on understanding the underlying concepts. Each exercise builds on previous knowledge, ensuring a well-rounded comprehension of how exponents work and interact in different mathematical contexts.
The journey through exponents is one of discovery and efficiency. By engaging with these worksheets, students can:
- Gain confidence in applying basic and advanced exponent rules
- Improve their ability to simplify complex expressions
- Understand the relationship between exponents and logarithms
- Handle scientific notation with ease
In summary, mastering exponent operations opens up a world of mathematical simplicity and elegance. From understanding how to multiply and divide powers to recognizing the importance of negative and zero exponents, these worksheets provide a structured path to proficiency. Whether for schoolwork, standardized tests, or personal enrichment, the practice offered here will undoubtedly elevate one's mathematical prowess.
What are some common mistakes when dealing with exponents?

+
One common mistake is not properly understanding that ( a^m \times a^n \neq a^{m \times n} ). Another is misunderstanding the sign of exponents when moving numbers between the numerator and denominator.
How do exponents help in solving real-world problems?

+
Exponents are used in various real-world applications like calculating population growth, radioactive decay, financial compound interest, and scaling in computer science.
Can you learn logarithms without understanding exponents?

+
Understanding exponents is crucial because logarithms are essentially the inverse operation of exponentiation. You need a solid foundation in exponents to make sense of logarithms.
What’s the difference between a positive and negative exponent?

+
A positive exponent indicates repeated multiplication, whereas a negative exponent indicates division by that number raised to the positive power.