5 Easy Steps to Master 6th Grade Inequalities
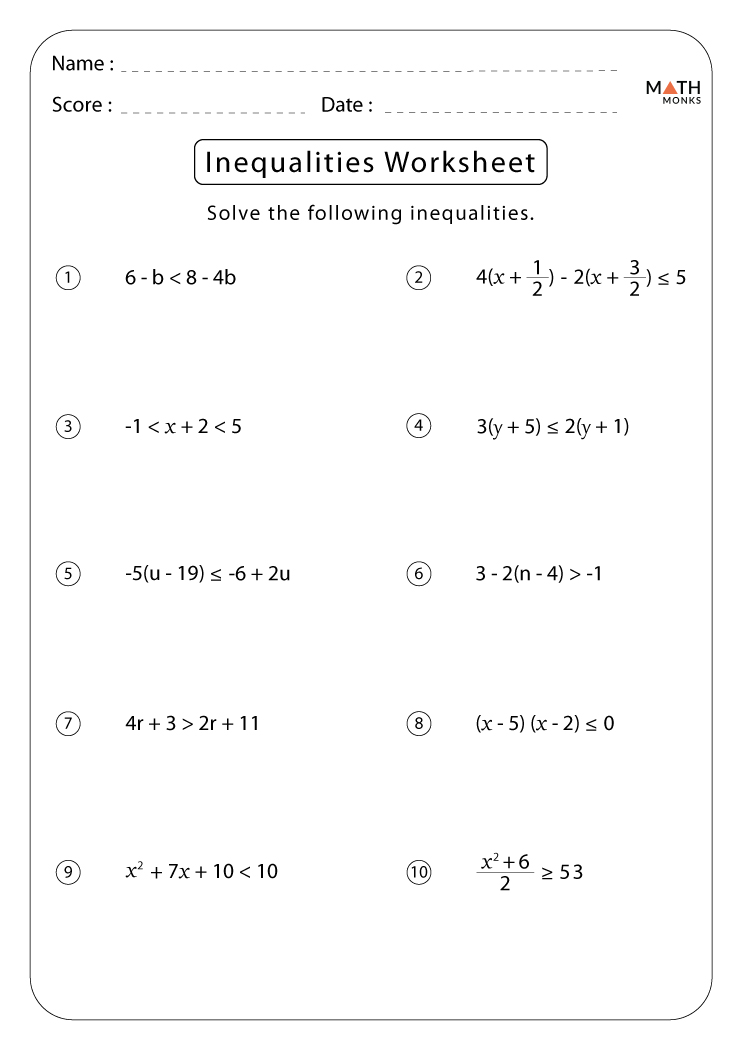
Learning to handle inequalities can seem challenging at first, but with the right approach, 6th graders can quickly grasp these mathematical concepts. Inequalities introduce students to the idea that two values or expressions aren't always equal, and this understanding is fundamental in real-world scenarios where exact equalities are rare. Here, we'll delve into five easy steps to help students master inequalities, providing them with tools to excel in math.
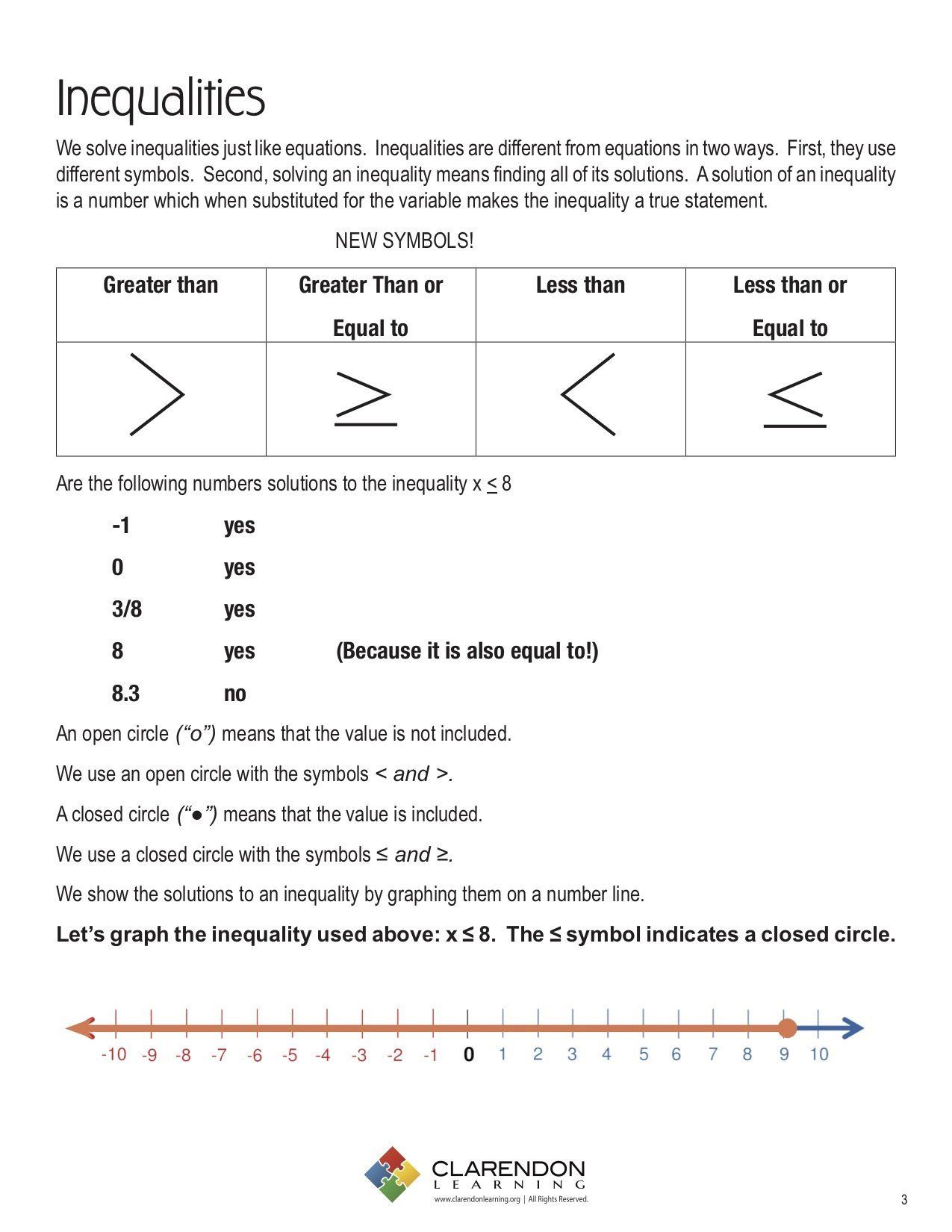
Step 1: Understanding Basic Inequality Symbols

Before diving into the mathematical operations, students need to recognize the basic inequality symbols:
- < - Less than
- > - Greater than
- ≤ - Less than or equal to
- ≥ - Greater than or equal to
It might help to relate these symbols to everyday comparisons. For example, “The number of cookies left after eating 3 from a box of 10 is less than or equal to 7.” This can be expressed as: 3 + x ≤ 10, where x represents the number of cookies eaten.
Step 2: Solving Simple Inequalities

Once students know the symbols, they can start solving simple inequalities:
- Identify the inequality sign and the direction of the solution.
- Isolate the variable by performing operations (addition, subtraction, multiplication, division) on both sides to balance the inequality.
- Remember that when you multiply or divide by a negative number, the inequality sign flips.
For instance, to solve the inequality 2x + 3 < 11:
- Subtract 3 from both sides: 2x < 8.
- Divide by 2: x < 4.
Here, we’ve balanced the equation by isolating x, ensuring that we keep the direction of the inequality consistent.
Step 3: Graphing Inequalities on a Number Line

Graphing inequalities visually represents the solutions set, which can be very helpful for visual learners:
- Use an open circle for < or >, indicating that the value is not included.
- Use a closed circle for ≤ or ≥, meaning the value is included.
- Extend the line to show the direction of the inequality.
For example, the inequality x > -3 would be represented by an open circle at -3, with a line extending to the right indefinitely.
💡 Note: The direction of the line tells you which values are part of the solution.
Step 4: Understanding Compound Inequalities

Students need to learn to tackle compound inequalities, where two inequalities are joined by “and” or “or”:
- With “and,” values must satisfy both inequalities, creating an interval between two numbers.
- With “or,” values can satisfy either one inequality or the other.
For example, for -1 < x ≤ 4:
- This is an “and” compound inequality, showing that x must be between -1 and 4, inclusive of 4.
- The graph would have an open circle at -1, a closed circle at 4, and a solid line connecting them.
Step 5: Solving Word Problems Involving Inequalities

Real-world applications help cement the understanding of inequalities:
- Translate the problem into an inequality.
- Solve the inequality for the variable.
- Interpret the results in the context of the problem.
Here’s a simple example:
- A store has fewer than 15 candles left. If they already sold 10 candles, write an inequality to find how many candles are left. The answer would be x < 15 - 10, which simplifies to x < 5. There are fewer than 5 candles left.
In summary, mastering inequalities is not just about solving equations; it's about understanding how numbers relate to each other in non-exact ways. By starting with the basic symbols, practicing simple solutions, graphing, handling compound inequalities, and applying these concepts to word problems, students can build a robust understanding of this important math topic. The practical applications of inequalities make it not just a theoretical exercise, but a skill useful for decision-making and problem-solving in everyday life.
What’s the difference between an equation and an inequality?
+
An equation states that two expressions are equal, using an equal sign (=). An inequality, on the other hand, expresses a relationship where one side is not necessarily equal to the other, using symbols like <, >, ≤, or ≥.
How do you know when to use < or ≤?

+
Use < when the boundary value is not included in the solution set. Use ≤ when the boundary value is included. For example, x < 5 means x can be any number less than 5, but not 5 itself; x ≤ 5 means x can be 5 or any number less than 5.
Why does the inequality sign flip when multiplying or dividing by a negative?

+
When you multiply or divide both sides of an inequality by a negative number, you reverse the order of the numbers relative to each other. This change in order necessitates a flip in the inequality sign to preserve the original relationship.
Can compound inequalities have no solutions or all real numbers as solutions?

+
Yes, a compound inequality can have no solutions if the conditions contradict each other (like x > 3 and x < 3). Conversely, it can be true for all real numbers if the conditions overlap completely (like x ≤ 5 and x > -∞).