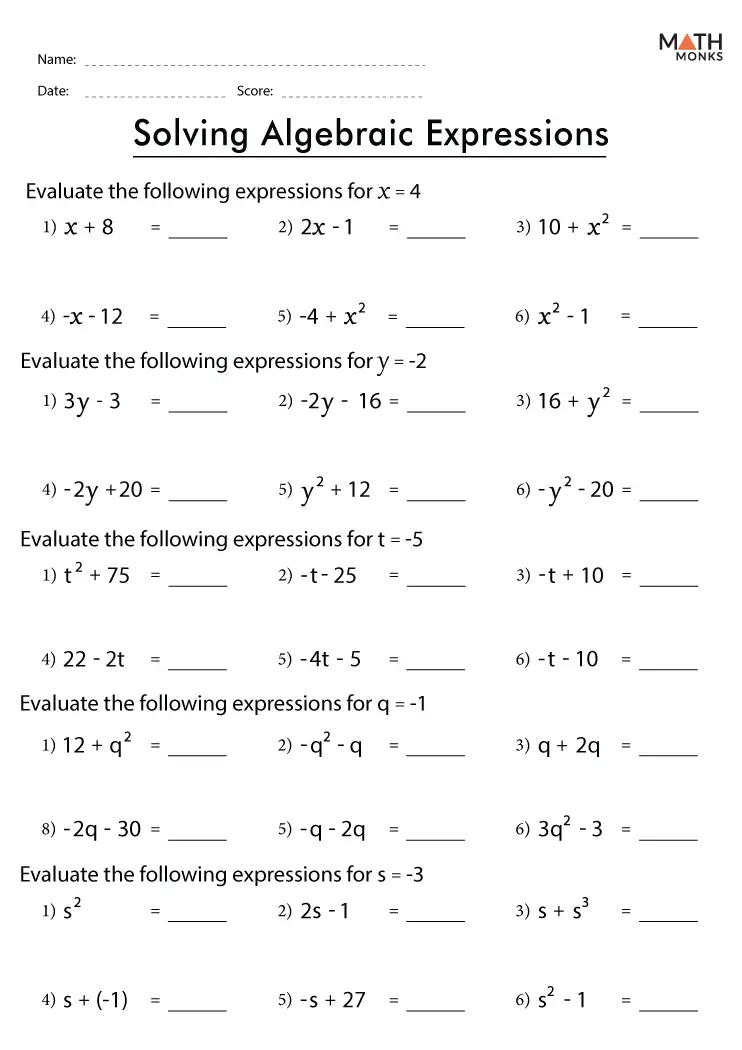
6 Ways to Master Algebraic Expressions for 6th Graders

In the fascinating world of mathematics, algebraic expressions form a foundational block that middle school students must conquer. For 6th graders embarking on this journey, understanding algebraic expressions is not just about solving equations; it's about developing logical thinking, problem-solving skills, and a deep-seated appreciation for the abstract beauty of numbers. Here are six foolproof strategies designed specifically for young mathematicians to master algebraic expressions:
1. Start with the Basics: Variables and Coefficients

The first step in understanding algebraic expressions is to familiarize oneself with the variables and coefficients:
- Variables are symbols that represent unknown values, typically denoted by letters like x, y, z.
- Coefficients are the numerical factors that multiply these variables.
For example, in the expression 4x, the number 4 is the coefficient, and x is the variable.
2. Visualize Algebraic Concepts

Mathematical understanding often benefits from visualization:
- Use algebra tiles or balance scales to physically represent equations.
- Create charts or diagrams to show how expressions change when variables are added, subtracted, multiplied, or divided.
3. Make It Fun with Real-Life Examples
Math isn't just in textbooks; it's everywhere around us:
- Explain how algebra can be used to solve daily puzzles like calculating the total cost of several items or determining the best deals when shopping.
- Use scenarios like the age difference between siblings (Age of John + Age of Mary = 15) to introduce variables and equations.
4. Practice Simplification and Expansion

The core of algebra lies in simplifying and expanding expressions:
- Start with basic expressions like 2x + 3x to show that 5x results from combining like terms.
- Use distributive property for expressions like 3(2x + 1) which expands to 6x + 3.
🧠 Note: Simplification and expansion are key skills that students will need for more advanced math topics.
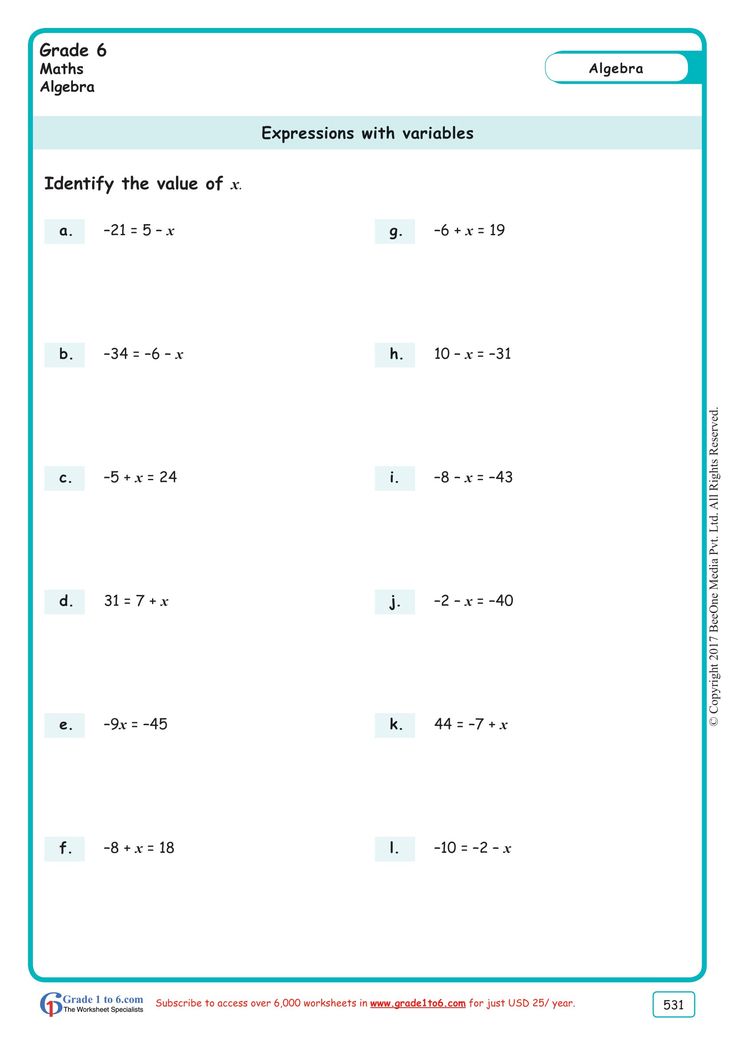
5. Solve Simple Equations

Once students are comfortable with expressions, introduce solving simple equations:
- Set up equations using word problems, which encourages problem-solving skills.
- Guide students through the process of isolating variables step-by-step.
6. Use Technology and Online Resources

There is a wealth of online tools and games designed to make algebra fun:
- Interactive algebra games can turn learning into play, reinforcing concepts through repetition and fun.
- Websites and apps like Khan Academy or IXL provide lessons and practice problems tailored to different learning levels.
Algebra might seem daunting at first, but with these six strategies, 6th graders can unlock its secrets. By focusing on foundational concepts, employing visual aids, making real-world connections, practicing key operations, solving problems, and leveraging technology, students can not only master algebraic expressions but also develop a love for this magical field of numbers. As they progress, they'll realize that algebra isn't just a set of operations to follow but a language that describes relationships and changes in the world around them, opening doors to higher mathematical understanding and problem-solving abilities.
What is a variable in algebra?

+
A variable is a symbol, often a letter, used to represent an unknown or changing value in algebraic expressions and equations. It can represent different quantities depending on the context.
How can I help my child understand algebra better?

+
Encourage your child with real-life examples, use visual aids, practice with them, and consider using educational tools online that make learning algebra interactive and fun.
Why is it important for 6th graders to learn algebra?

+
Algebra forms the foundation for higher mathematics. Learning algebra at an early age helps students develop logical reasoning, critical thinking, and problem-solving skills which are essential in many areas of life and academics.
What are common mistakes students make when learning algebra?
+
Common mistakes include misunderstanding the difference between variables and constants, forgetting to distribute when expanding expressions, and errors in combining like terms or performing operations in the wrong order.