5th Grade Math Fun: Order of Operations Worksheets

In the world of mathematics, mastering the order of operations is fundamental for understanding complex calculations. This skill is typically introduced in 5th grade math, where students learn the sequence in which they should perform mathematical operations. Not only does this pave the way for algebra and higher mathematics, but it also helps in real-life problem-solving where calculations are involved. Here's how you can make learning order of operations fun and effective through engaging worksheets.
Why Order of Operations is Important

The order of operations, often remembered by the acronym PEMDAS (Parentheses, Exponents, Multiplication and Division (from left to right), Addition and Subtraction (from left to right)), ensures that mathematical expressions have a consistent and unambiguous way of being evaluated. Here’s why it matters:
- Predictability and Clarity: Knowing the correct order avoids confusion and errors when solving problems.
- Foundational for Algebra: Algebraic expressions follow these rules, making them easier to understand and manipulate.
- Real-World Applications: From budgeting to understanding scientific formulas, the order of operations is used daily.
Creating Engaging Worksheets

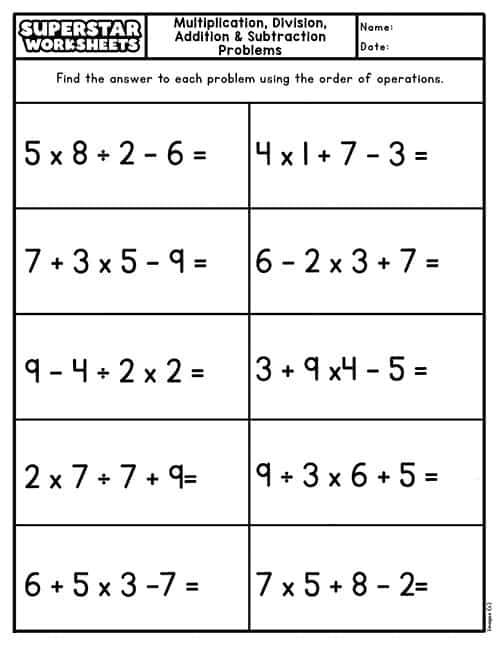
Here are several ideas for worksheets that can make learning the order of operations engaging:
1. Story Problem Puzzles

Create puzzles where students must solve for quantities using order of operations. Here’s an example:
| Problem | Solution |
|---|---|
| Jeff bought 3 video games. Each game cost 20, but he also got a 10% discount on one of them. How much did he spend in total?</td> <td>Step 1: Calculate the cost of one game after discount (20 - 20 * 0.10 = 18). Step 2: Multiply the cost of two games without discount by 2 (20 * 2 = 40). Step 3: Add them together (40 + 18 = 58). |

📝 Note: Ensure the problems are age-appropriate and the discounts or quantities make sense in a real-life context.
2. Operation Hunt

Construct sentences where students must find hidden mathematical operations. For example:
- “When the clown was juggling 5 balls, he dropped 3 and picked up 1 again. How many balls does he have now?”
3. Fill in the Blanks

Provide an equation where operations are missing, and students must fill them in:
- 2 __ 6 + 3 = 17
- They should figure out it’s 2 * 6 + 3 = 17.
4. Visual Mathematics
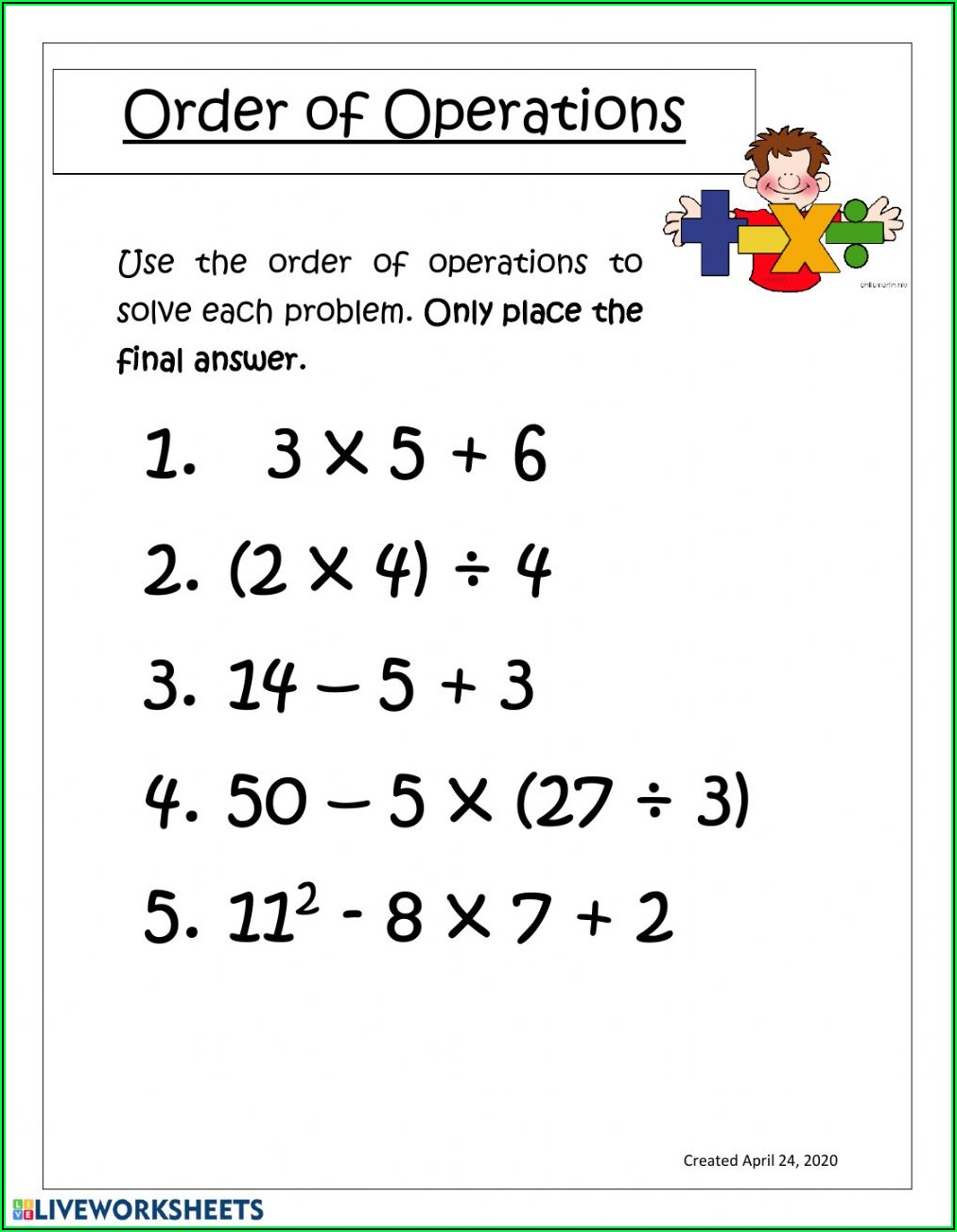
Use diagrams or pictures where students can “see” the operations:

In this image, students would add the apples first, then subtract the apples placed in the basket.
How to Use These Worksheets Effectively

To ensure your worksheets are effective:
- Start Simple: Begin with straightforward equations to build confidence.
- Gradual Complexity: Increase the complexity as students master the basics.
- Engage with Stories: Use relatable scenarios or funny situations to keep children interested.
- Review and Discuss: After each worksheet, go over the solutions together to clarify misunderstandings.
- Encourage Group Work: Students often learn better when they explain concepts to each other.
In weaving the order of operations into 5th grade math education, the goal is not just to teach a rule but to instill a logical approach to problem-solving. By making the learning process enjoyable and relevant, we foster a love for math that can last a lifetime. Students equipped with this foundational knowledge will find their path through higher mathematics much smoother. Remember, patience and practice are key.
What is the PEMDAS rule?

+
The PEMDAS rule stands for Parentheses, Exponents, Multiplication and Division (from left to right), and Addition and Subtraction (from left to right). This acronym helps students remember the correct order to solve mathematical expressions.
Why do we need the order of operations?

+
The order of operations ensures that all mathematicians solve an expression in the same way, reducing ambiguity and error in calculations. It’s crucial for consistency and accuracy in mathematics.
Can order of operations be applied outside of school?
+
Yes, the order of operations is not just for classroom math. It’s used in everyday situations like budgeting, calculating discounts, understanding scientific formulas, and even in computer programming where logic and sequencing are critical.