5 Answers to Parallel Lines and Transversals Worksheet

Understanding Parallel Lines and Transversals

Parallel lines and transversals form one of the foundational concepts in geometry, particularly in the study of angles and lines. A transversal is a line that crosses at least two other lines. When a transversal intersects two parallel lines, it creates several pairs of angles with specific relationships. In this detailed exploration, we will dive into the intricacies of these angle relationships, offer step-by-step guidance on solving problems related to parallel lines and transversals, and equip you with tools to ace your worksheet exercises.
Types of Angles Formed
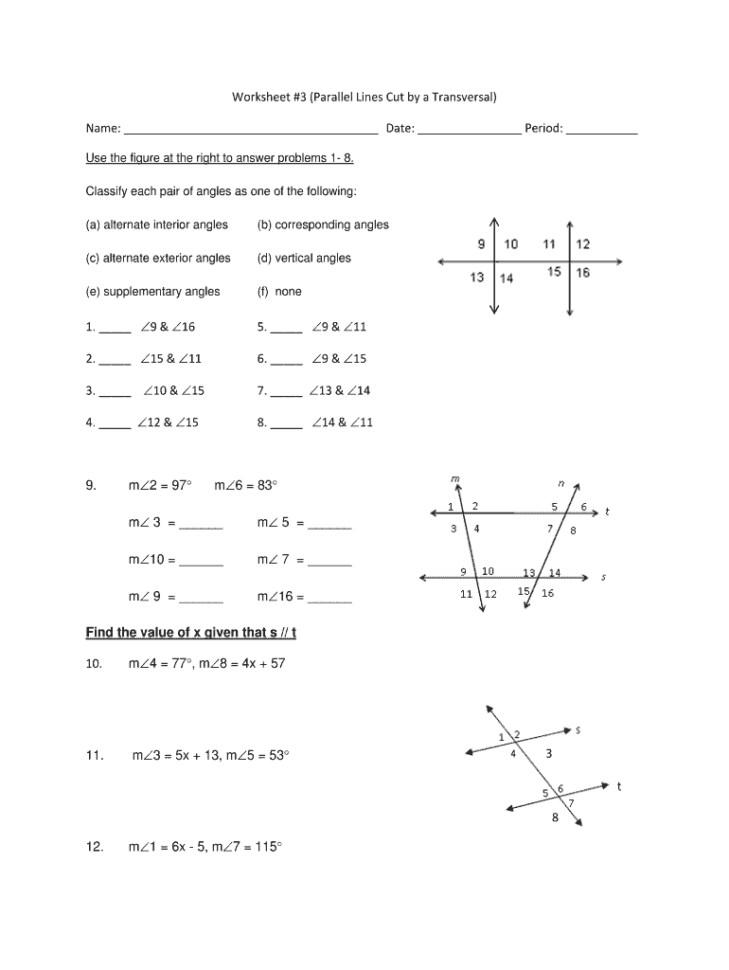
When a transversal intersects parallel lines, the angles formed can be categorized into:
- Corresponding angles - Angles that are in the same relative position on both lines.
- Alternate interior angles - Angles that are inside the parallel lines and on opposite sides of the transversal.
- Alternate exterior angles - Angles that are outside the parallel lines and on opposite sides of the transversal.
- Consecutive interior angles (or same-side interior angles) - Angles that are inside the parallel lines and on the same side of the transversal.
| Angle Pair | Relationship | Symbol |
|---|---|---|
| Corresponding Angles | Equal (congruent) | ∠1 ≅ ∠5, ∠2 ≅ ∠6, ∠3 ≅ ∠7, ∠4 ≅ ∠8 |
| Alternate Interior Angles | Equal (congruent) | ∠3 ≅ ∠6, ∠4 ≅ ∠5 |
| Alternate Exterior Angles | Equal (congruent) | ∠1 ≅ ∠8, ∠2 ≅ ∠7 |
| Consecutive Interior Angles | Supplementary (sum to 180°) | ∠3 + ∠5 = 180°, ∠4 + ∠6 = 180° |

Step-by-Step Problem Solving

To solve problems involving parallel lines and transversals:
- Identify the transversal - This will help you understand which angles are related.
- Label your angles - Use consistent labels to avoid confusion.
- Determine the relationships - Based on the type of angle pair.
- Set up an equation - Use the relationships to form equations.
- Solve for the unknown angle - Use algebra to find the measures of the angles.
Example Problem
Given that lines l and m are parallel, find the value of x if ∠1 = (2x + 30)° and ∠5 = 110°.
- Since ∠1 and ∠5 are corresponding angles, they are equal when lines l and m are parallel.
- Set up the equation: 2x + 30 = 110.
- Solve for x:
- Subtract 30 from both sides: 2x = 80.
- Divide both sides by 2: x = 40.
💡 Note: Always verify your solution by substituting x back into the original problem to check if it fits all known angles.
Understanding the Geometry

Parallel lines and transversals not only involve the understanding of angle pairs but also require a good grasp of:
- The properties of parallel lines.
- Linear pairs and supplementary angles.
- How angles in triangles relate to this context.
Practical Applications
The study of parallel lines and transversals extends beyond geometry:
- In architecture for designing structures with precise angles.
- In navigation and cartography for determining bearings and trajectories.
- In computer graphics for rendering and perspective projection.
Real-world Examples
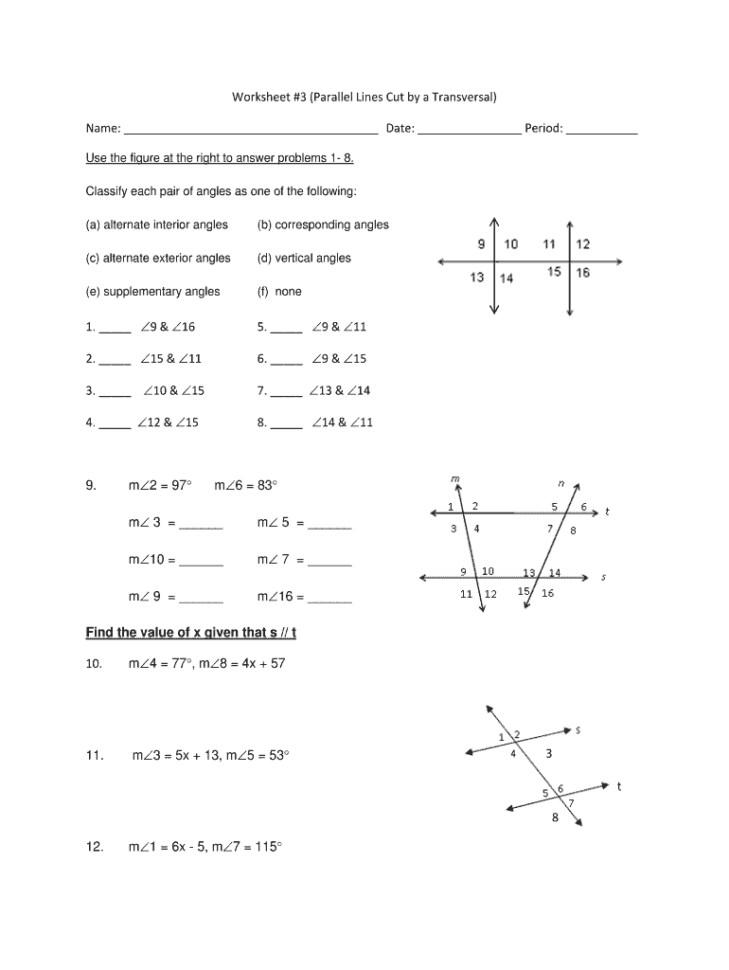
Let’s look at some real-world scenarios:
- Building design - The layout of a building can use angles formed by transversals intersecting parallel walls.
- Road signs - Highway signs often show angles that help drivers anticipate turns or intersections.
📐 Note: In practical applications, the angles need not be perfect due to human error and design considerations, but the principles remain the same.
In summary, understanding how parallel lines and transversals interact forms the backbone of much geometric analysis. The relationships among corresponding, alternate, and consecutive angles provide not only an academic foundation but also practical applications in various fields. By grasping these concepts, you can solve problems, design with precision, and appreciate the elegance of geometric symmetry and order.
What are corresponding angles?

+
Corresponding angles are angles that occupy the same relative position on each of two lines when intersected by a transversal. They are congruent in parallel lines.
How do I know if two lines are parallel?

+
Lines are parallel if they never intersect or if they form congruent corresponding, alternate interior, or alternate exterior angles when cut by a transversal.
Can I use transversals in three-dimensional space?

+
Yes, although the study becomes more complex due to additional planes and perspectives, the same principles apply regarding the angles formed.
What is the difference between parallel and perpendicular lines?

+
Parallel lines never intersect, whereas perpendicular lines intersect at a right angle (90°).
How can transversals be applied in engineering?

+
In engineering, transversals help in creating precise structures, like bridges or steel frames, where angles and alignments need to be exact for stability and safety.