5 Ways to Solve 2-Step Equations with Fractions
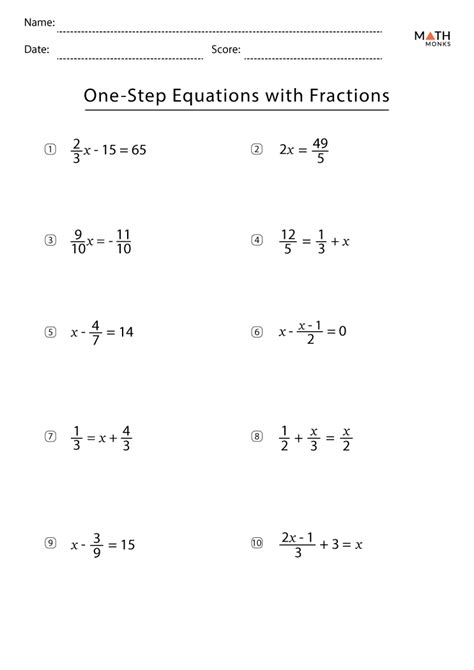
Understanding 2-Step Equations with Fractions

When solving linear equations, we often encounter problems that involve fractions. These equations can be a bit more challenging, but with the right strategies, you can solve them with ease. In this article, we will explore five ways to solve 2-step equations with fractions. Before we dive into the solutions, let’s first understand what 2-step equations are and how fractions work in equations.
What are 2-Step Equations?
A 2-step equation is a linear equation that requires two operations to solve. These operations can be addition, subtraction, multiplication, or division. The goal is to isolate the variable (usually x) on one side of the equation.
How Fractions Work in Equations
Fractions in equations can be added, subtracted, multiplied, or divided, just like whole numbers. However, when working with fractions, we need to follow the rules of fractions. For example, when multiplying or dividing fractions, we need to follow the rules of multiplying or dividing numerators and denominators.
Method 1: Inverting and Multiplying

One way to solve 2-step equations with fractions is by inverting and multiplying. This method involves multiplying both sides of the equation by the reciprocal of the fraction.
Example: Solve for x in the equation 1/2x + 3 = 7
To solve this equation, we need to isolate x. First, we subtract 3 from both sides:
1/2x = 7 - 3 1/2x = 4
Next, we multiply both sides by the reciprocal of 1⁄2, which is 2:
x = 4 × 2 x = 8
Notes:
- Make sure to multiply both sides of the equation by the reciprocal of the fraction to avoid changing the equation’s value.
- This method works best when the fraction is on the same side as the variable.
Method 2: Using the LCD
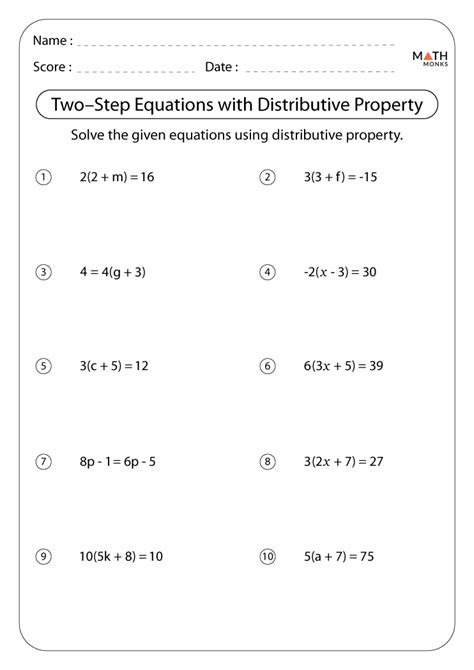
Another method to solve 2-step equations with fractions is by using the Least Common Denominator (LCD). This method involves finding the LCD of the fractions and multiplying both sides of the equation by the LCD.
Example: Solve for x in the equation 1/4x + 1⁄2 = 3⁄4
To solve this equation, we need to find the LCD of the fractions, which is 4. Next, we multiply both sides of the equation by 4:
4(1/4x) + 4(1⁄2) = 4(3⁄4) x + 2 = 3
Now, we subtract 2 from both sides:
x = 3 - 2 x = 1
Notes:
- Finding the LCD can be challenging, especially when dealing with complex fractions.
- This method works best when there are multiple fractions in the equation.
Method 3: Simplifying Fractions

Simplifying fractions can also help solve 2-step equations. This method involves simplifying the fractions on both sides of the equation and then solving for the variable.
Example: Solve for x in the equation 2/4x + 1 = 3
To solve this equation, we can simplify the fraction 2⁄4 by dividing both the numerator and denominator by 2:
1/2x + 1 = 3
Next, we subtract 1 from both sides:
1/2x = 2
Now, we multiply both sides by the reciprocal of 1⁄2:
x = 2 × 2 x = 4
Notes:
- Simplifying fractions can make the equation easier to solve.
- This method works best when the fractions can be simplified easily.
Method 4: Using Equivalent Ratios

Using equivalent ratios can also help solve 2-step equations with fractions. This method involves creating an equivalent ratio by multiplying or dividing both sides of the equation by the same value.
Example: Solve for x in the equation 3/4x = 9⁄2
To solve this equation, we can create an equivalent ratio by multiplying both sides by 4:
3x = 9 × 2 3x = 18
Next, we divide both sides by 3:
x = 18 ÷ 3 x = 6
Notes:
- Using equivalent ratios can help simplify the equation.
- This method works best when the fractions are already in their simplest form.
Method 5: Eliminating Fractions by Adding or Subtracting
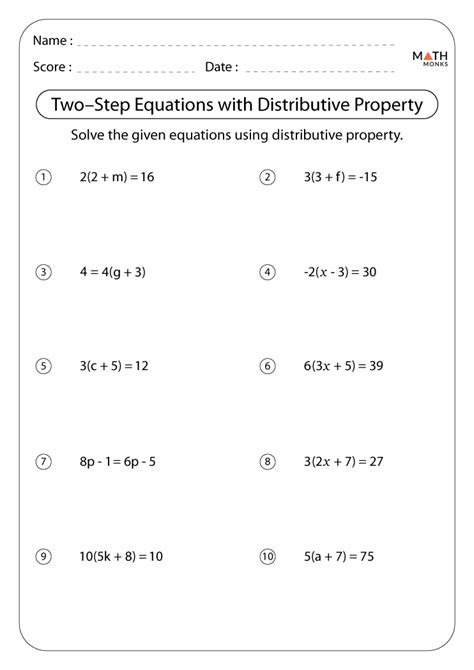
Finally, eliminating fractions by adding or subtracting can also solve 2-step equations. This method involves adding or subtracting a value to both sides of the equation to eliminate the fraction.
Example: Solve for x in the equation x - 1⁄3 = 2
To solve this equation, we can add 1⁄3 to both sides:
x = 2 + 1⁄3
Now, we can simplify the fraction:
x = 2 1⁄3
Notes:
- Eliminating fractions by adding or subtracting can be a quick way to solve equations.
- This method works best when the fraction is on the same side as the variable.
What is the best method to solve 2-step equations with fractions?
+
The best method depends on the specific equation. If the fraction is on the same side as the variable, inverting and multiplying may be the best method. If there are multiple fractions, using the LCD may be more effective.
How do I simplify fractions in an equation?

+
To simplify fractions, divide both the numerator and denominator by the greatest common divisor (GCD). For example, 2/4 can be simplified to 1/2 by dividing both the numerator and denominator by 2.
Can I use equivalent ratios to solve all types of 2-step equations with fractions?
+
No, equivalent ratios may not work for all types of 2-step equations with fractions. This method works best when the fractions are already in their simplest form and can be easily multiplied or divided.
In conclusion, solving 2-step equations with fractions requires a combination of mathematical strategies and techniques. By understanding how fractions work in equations and applying the right methods, you can solve even the most challenging equations. Remember to always simplify fractions, use equivalent ratios, and eliminate fractions by adding or subtracting to make the equation easier to solve. With practice and patience, you can master the art of solving 2-step equations with fractions.
Related Terms:
- two step equations worksheets pdf
- Multi Step Equations Worksheet
- two step equation worksheet pdf
- 2 step equations decimals worksheet
- two step equations pdf free
- worksheets solving two step equations