2 Step Equation Worksheets: Master Math Easily
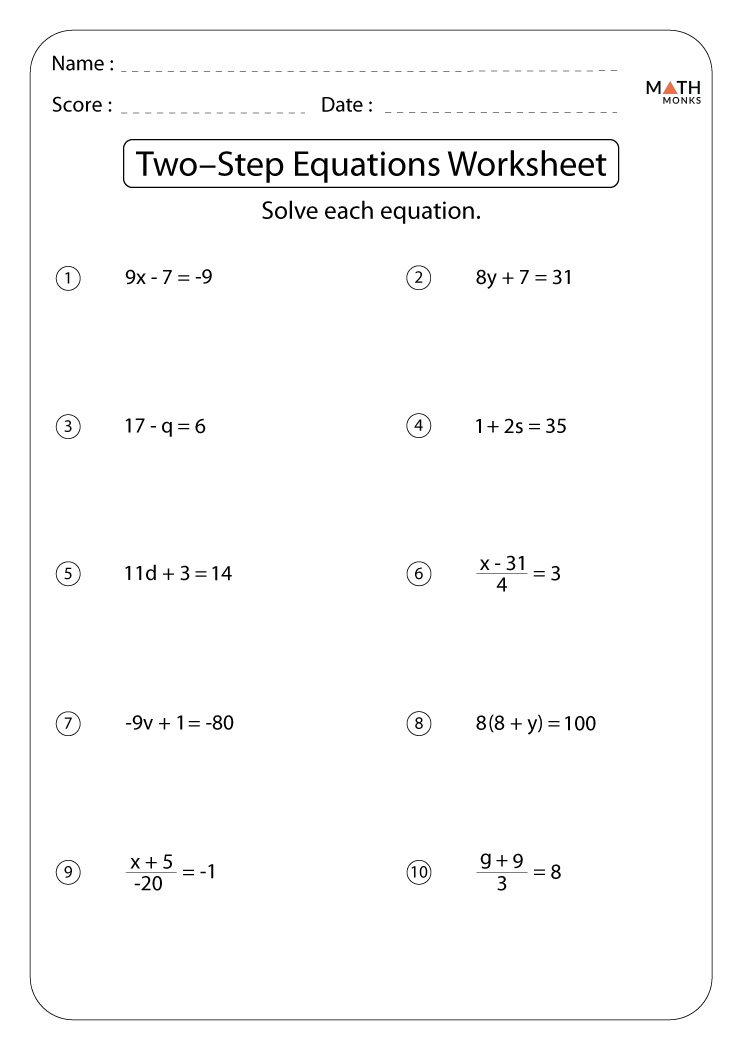
Understanding two-step equations is crucial for anyone aiming to master mathematics. These equations involve two operations, providing a stepping stone from basic arithmetic to more complex algebraic problems. In this guide, we'll explore the essence of two-step equations, provide worksheets, and deliver strategies to solve them efficiently.
Understanding Two-Step Equations
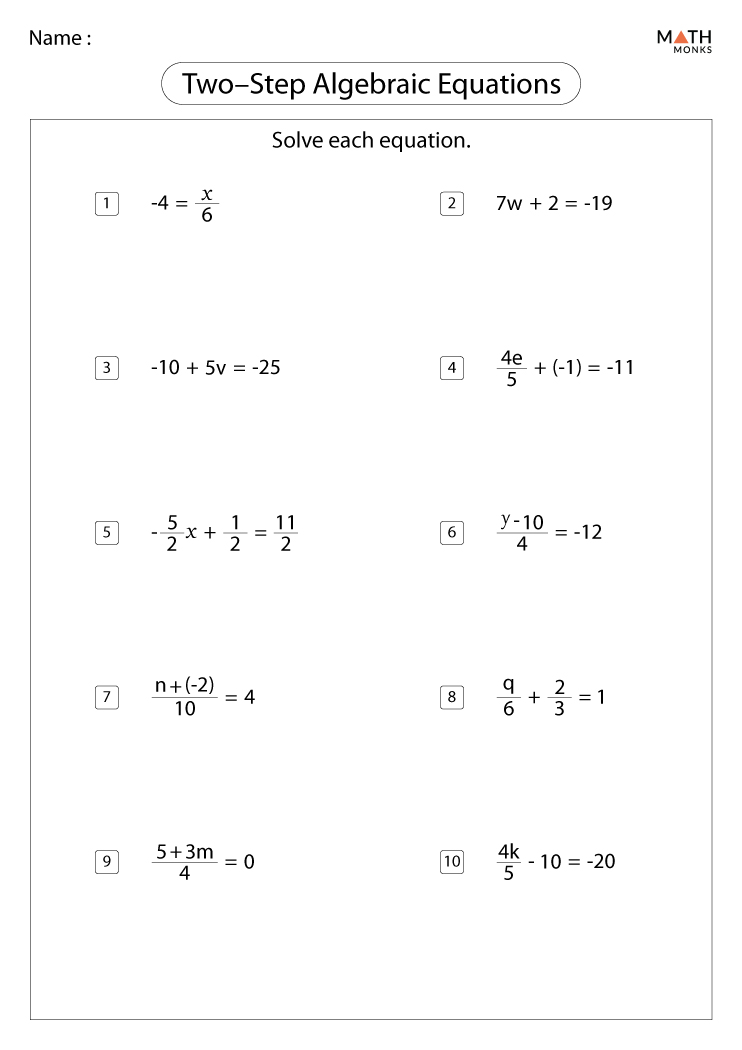
Before diving into the worksheets, let’s grasp what two-step equations are. They are algebraic expressions requiring two operations to isolate the variable, often (x). This typically involves:
- First, removing an added or subtracted term (by performing the inverse operation).
- Second, dealing with a term multiplied or divided with the variable (by division or multiplication).
Here’s a simple example:
[ 3x + 5 = 17 ]
To solve:
- Subtract 5 from both sides to get (3x = 12).
- Divide both sides by 3 to isolate (x), giving (x = 4).
Downloadable Two-Step Equation Worksheets

For mastering these equations, nothing beats practice. Here are some benefits of using worksheets:
- Provides structured practice in solving two-step equations.
- Allows for repetition, which is key to understanding.
- Offers various levels of difficulty to cater to different learning stages.
| Worksheet Name | Description | Difficulty Level |
|---|---|---|
| Beginner’s Delight | Introduces basic two-step equations with positive integers. | Beginner |
| Middle Ground | Includes negative numbers and more complex calculations. | Intermediate |
| Algebra Aces | Features variables on both sides, fractions, and decimals. | Advanced |

Strategies for Solving Two-Step Equations

Mastering two-step equations involves more than just practice. Here are some effective strategies:
- Understand the Operations: Identify the operations first. Addition or subtraction? Multiplication or division?
- Isolate the Variable: Work on one side of the equation to isolate the variable by performing inverse operations.
- Check Your Work: Once you’ve solved for (x), plug the value back into the original equation to verify your solution.
⚠️ Note: Always double-check your steps and the final solution by substituting x back into the equation.
Tips for Effective Learning

Here are some additional tips to help you or your students master two-step equations:
- Explain the reasoning behind each step to reinforce understanding.
- Use visual aids or algebraic tiles to represent equations physically.
- Encourage students to verbalize their thought processes during problem-solving.
- Discuss real-world applications of two-step equations to make learning relevant.
Recap

Mastering two-step equations is about understanding the steps, practicing regularly with varied worksheets, and using effective strategies to solve equations systematically. By recognizing the operations, isolating variables, and verifying solutions, students can become proficient in solving these equations. Use the provided worksheets for practice, and the strategies outlined here to guide your learning journey or that of your students.
What is the importance of understanding two-step equations in mathematics?

+
Two-step equations are fundamental in algebra. They teach the principles of isolating variables, which is crucial for tackling more complex algebraic problems and equations with more than two steps.
Can two-step equations be applied to real-life situations?

+
Absolutely! From calculating taxes to budgeting, two-step equations help in understanding how different variables interact, allowing for practical problem-solving in daily life.
What if I get stuck while solving a two-step equation?

+
Don’t get discouraged. Review your steps, ensure you’ve performed the correct inverse operations, and sometimes, stepping away for a moment and coming back with a fresh perspective can help.
How do I know if my solution to a two-step equation is correct?

+
Substitute the value you found for (x) back into the original equation. If both sides balance out, your solution is correct. This is a great habit to develop for all algebraic problems.