5 Essential Tips for Mastering 2-Step Algebra Equations

2-step algebra equations are the building blocks of understanding how to solve more complex equations in math. They require a careful, step-by-step approach to ensure accuracy and understanding. Let's delve into five essential tips that will not only help you master 2-step algebra equations but also make them fun and engaging.
1. Understanding the Basics

Before diving into 2-step algebra equations, ensure you are comfortable with the following:
- Order of Operations - Understand PEMDAS (Parentheses, Exponents, Multiplication and Division from left to right, Addition and Subtraction from left to right).
- Variables and Constants - Know that variables represent unknown numbers, and constants are fixed values.
- Basic Operations - Have a firm grip on addition, subtraction, multiplication, and division.
🔍 Note: Mastering these basics will make tackling 2-step algebra equations significantly easier.
2. Isolate the Variable

The golden rule in solving algebra equations is to isolate the variable. For 2-step equations, this often means:
- Removing terms on the side of the variable using the inverse operation. If there's addition or subtraction next to the variable, subtract or add the same value on both sides to eliminate it.
- Using multiplication or division to extract the variable's value. Multiply or divide both sides of the equation by the same number to get the variable by itself.
Here's an example:
To solve for x in the equation 2x + 3 = 11:
- Subtract 3 from both sides:
2x = 8 - Divide both sides by 2:
x = 4
🧩 Note: Remember to always apply the same operation to both sides of the equation to keep it balanced.
3. Use the Inverse Operation
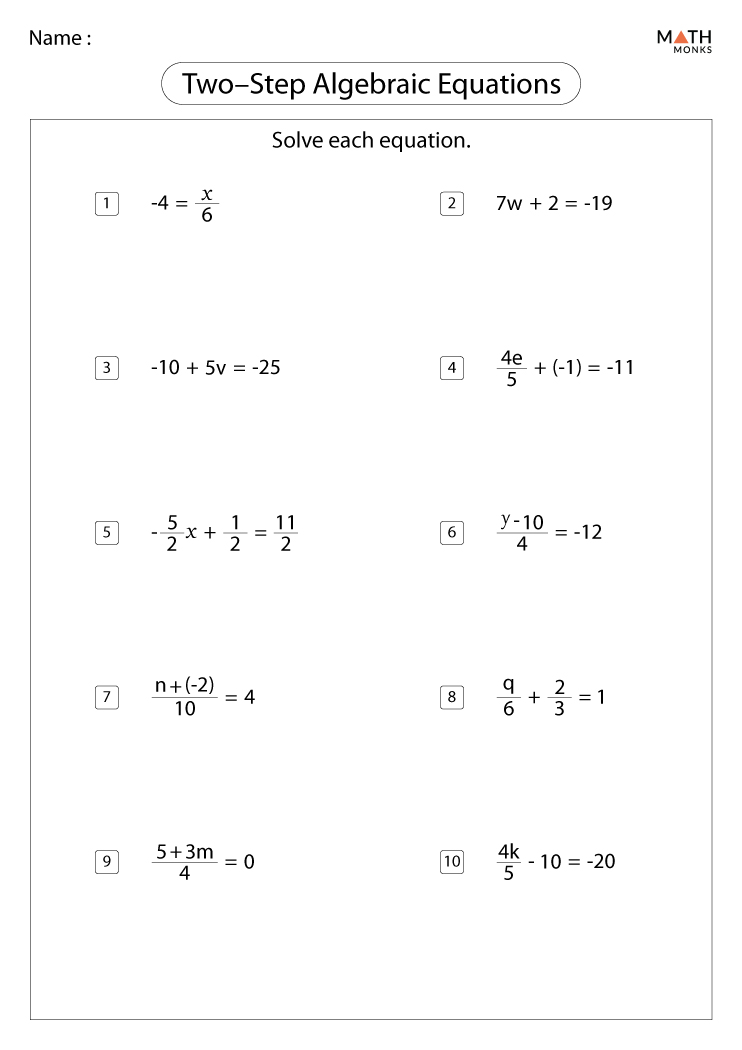
The key to solving 2-step algebra equations is to use inverse operations. Here are some common pairs:
| Operation | Inverse Operation |
|---|---|
| Addition | Subtraction |
| Subtraction | Addition |
| Multiplication | Division |
| Division | Multiplication |
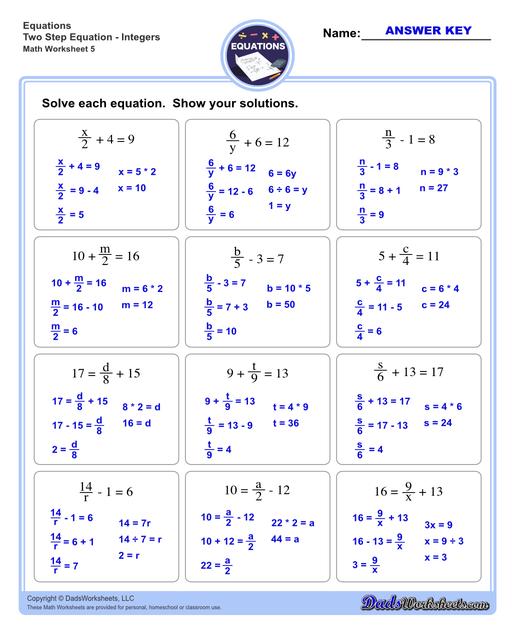
By undoing each step, you can isolate the variable, as shown in the previous example. Here’s another example for clarity:
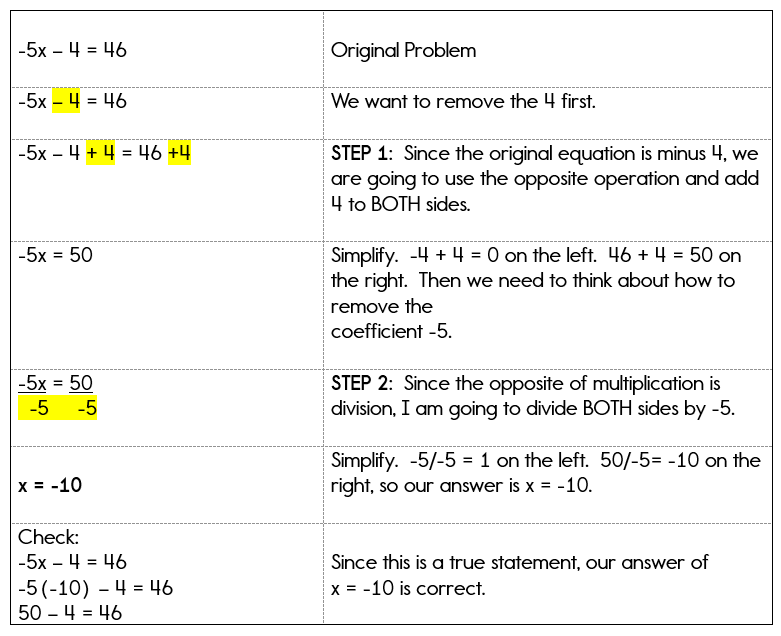
Solve 3x - 5 = 1:
- Add 5 to both sides:
3x = 6 - Divide both sides by 3:
x = 2
It's crucial to perform these steps in the correct order to maintain the integrity of the equation.
4. Checking Your Work
Once you've solved for the variable, it's beneficial to check your work. Here's how:
- Substitute the value you found back into the original equation.
- Perform the operations on both sides to verify if the equation is true.
In the equation 3x - 5 = 1, if you found x = 2:
- Substitute
x = 2:3(2) - 5 = 6 - 5 = 1 - Since the left side equals the right side, your solution is correct.
5. Practice Makes Perfect
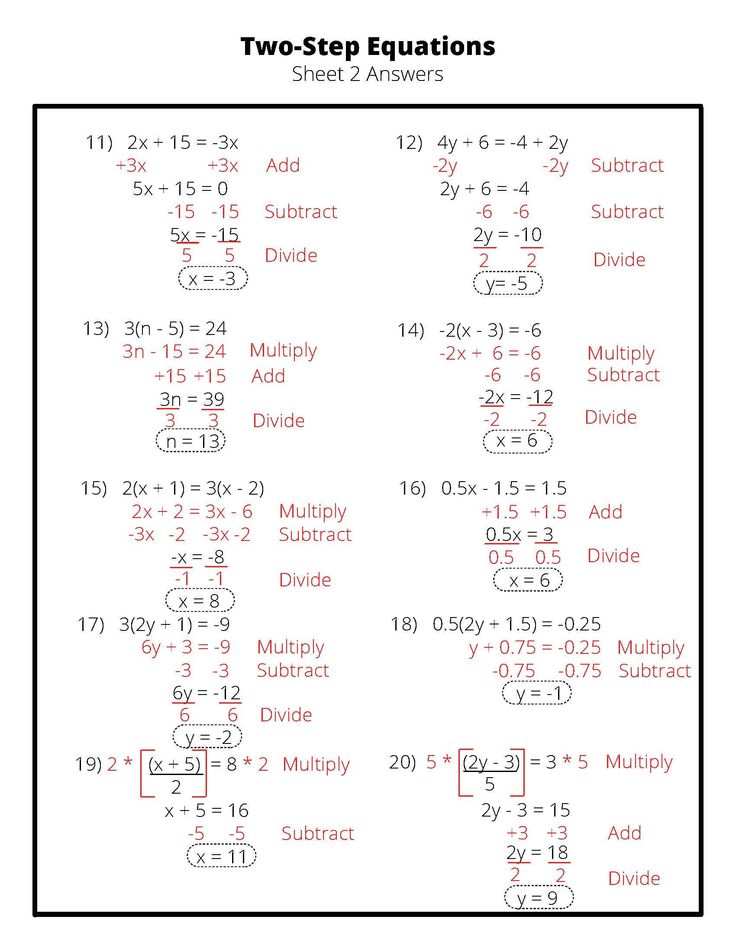
The final tip for mastering 2-step algebra equations is practice. Here are some ways to practice:
- Use online resources or apps for interactive problems.
- Work through a textbook or workbook.
- Form a study group for mutual support and learning.
📚 Note: Consistent practice will ingrain these steps, making solving equations almost intuitive.
In this journey of learning 2-step algebra equations, you've covered fundamental techniques, understood how to isolate variables using inverse operations, emphasized the importance of checking work, and recognized the critical role of practice. As you apply these strategies, you'll find yourself more adept at solving not just 2-step equations but also more complex algebraic problems. Remember, the key is not just to solve equations but to understand the logic behind each step, enhancing your problem-solving skills in mathematics and beyond.
Why do I need to isolate the variable in algebra?
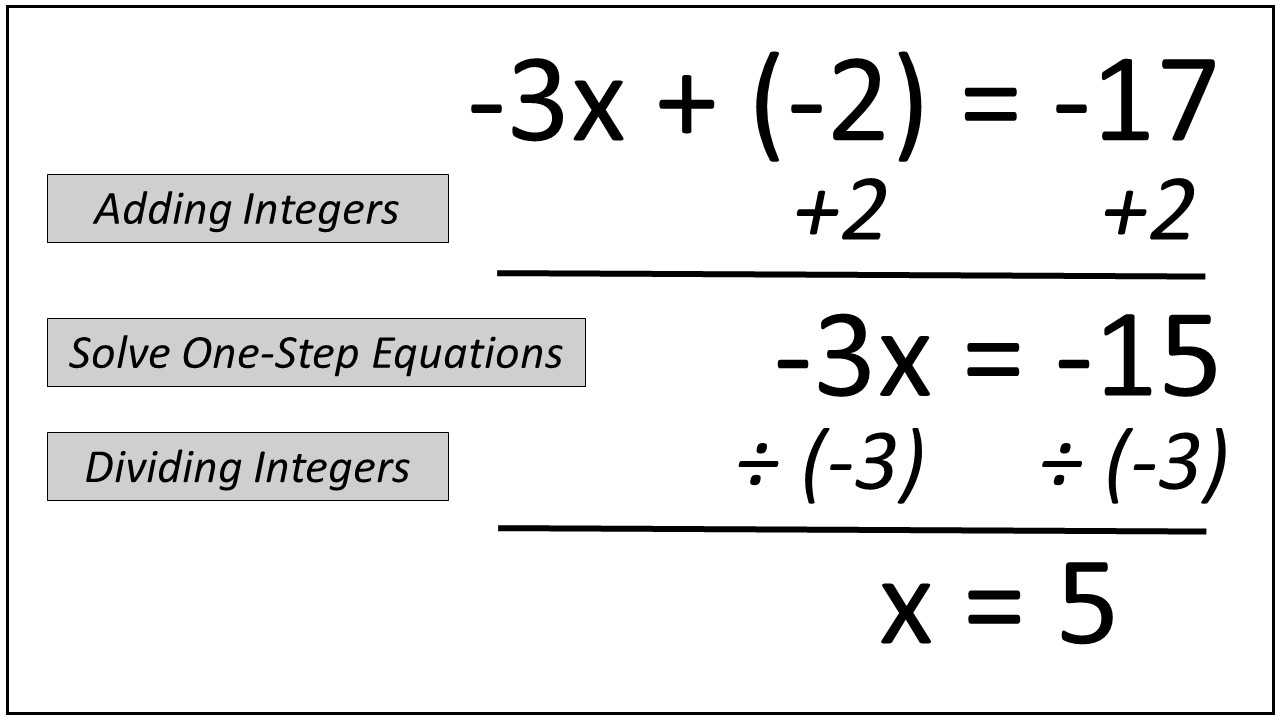
+
Isolating the variable allows you to determine its specific value or relationship within the equation, which is the primary goal in algebra.
Can I solve an equation by only applying one operation?

+
Typically, you’ll need to apply multiple operations to fully solve an equation. However, simpler equations might require just one step.
Is checking my work really necessary after solving an equation?

+
Yes, checking your work ensures accuracy and helps in building confidence in your problem-solving skills. It’s a valuable practice for all levels of mathematics.
How can I make algebra practice more fun?

+
Incorporate games, use interactive learning platforms, or try real-world applications of algebra to make practice more engaging.
What should I do if I get stuck on an algebra equation?
+
Take a break, review your steps, look for online tutorials or worked examples, or ask for help from a teacher, tutor, or study group.