5 Ways to Master 2 Digit by 2 Digit Multiplication

Mastering 2 Digit by 2 Digit Multiplication: A Comprehensive Guide
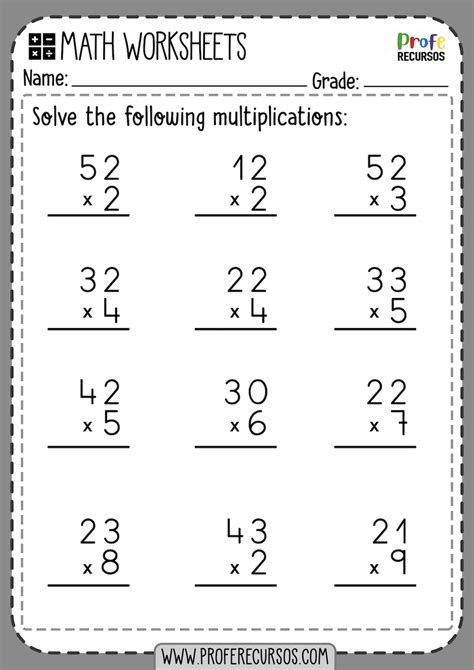
Multiplication is an essential arithmetic operation that we use daily, and mastering 2 digit by 2 digit multiplication is a crucial skill for students and professionals alike. In this article, we will explore five effective ways to master 2 digit by 2 digit multiplication, along with tips and tricks to make the process easier and more efficient.
Method 1: The Standard Algorithm

The standard algorithm for 2 digit by 2 digit multiplication involves multiplying the multiplicand (the number being multiplied) by each digit of the multiplier (the number by which we are multiplying), and then adding up the partial products. This method is widely used and is an excellent way to develop your multiplication skills.
For example, let’s multiply 43 by 27 using the standard algorithm:
43
x 27
------
43 x 20 = 860
43 x 7 = 301
------
860 + 301 = 1161
📝 Note: Make sure to line up the numbers correctly and perform the calculations accurately to avoid errors.
Method 2: The Lattice Method
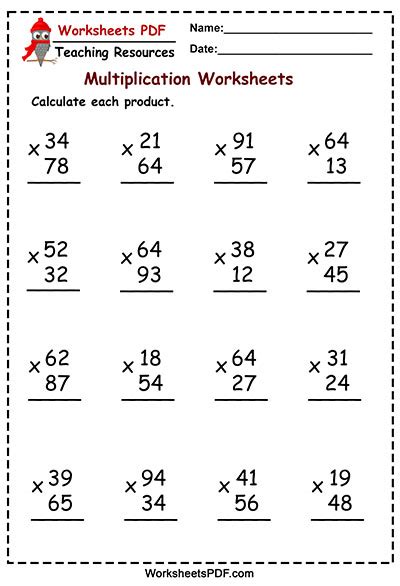
The lattice method is a visual approach to multiplication that involves creating a grid of numbers and using it to calculate the product. This method is particularly useful for students who struggle with the standard algorithm.
Here’s an example of how to use the lattice method to multiply 43 by 27:
| 20 | 7 | |
|---|---|---|
| 40 | 800 | 280 |
| 3 | 60 | 21 |
| — | — | — |
| 860 | 301 | |
| 1161 |

📝 Note: The lattice method can be time-consuming, but it's an excellent way to develop your multiplication skills and understand the concept of place value.
Method 3: The Partial Products Method
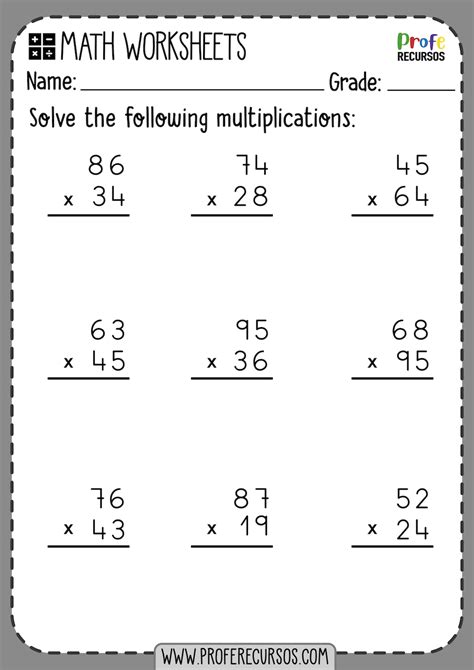
The partial products method involves breaking down the multiplication problem into smaller parts and calculating the product of each part separately. This method is similar to the standard algorithm, but it’s more visual and can be easier to understand.
For example, let’s multiply 43 by 27 using the partial products method:
- 40 x 20 = 800
- 40 x 7 = 280
- 3 x 20 = 60
- 3 x 7 = 21
Now, add up the partial products:
800 + 280 = 1080 1080 + 60 = 1140 1140 + 21 = 1161
📝 Note: The partial products method can be more time-consuming than the standard algorithm, but it's an excellent way to develop your understanding of place value and multiplication.
Method 4: The Nines Trick

The nines trick is a clever way to multiply numbers that end in 9. This method involves using the fact that 9 is equal to 10 - 1, and then using this fact to simplify the multiplication problem.
For example, let’s multiply 43 by 19 using the nines trick:
- 43 x 10 = 430
- 43 x -1 = -43
Now, add up the partial products:
430 - 43 = 387
📝 Note: The nines trick only works for numbers that end in 9, but it's an excellent way to simplify multiplication problems and develop your mental math skills.
Method 5: Mental Math Strategies

Mental math strategies involve using clever techniques to multiply numbers quickly and accurately. One popular strategy is the “double and double” method, which involves doubling the multiplicand and then doubling the multiplier.
For example, let’s multiply 43 by 27 using the double and double method:
- Double 43 = 86
- Double 27 = 54
Now, multiply 86 by 54:
- 86 x 50 = 4300
- 86 x 4 = 344
Add up the partial products:
4300 + 344 = 4644
However, this is not the correct answer. To get the correct answer, we need to divide 4644 by 2 (since we doubled both numbers):
4644 ÷ 2 = 2322
Then, add 21 (since 43 x 7 = 301, and 301 - 280 = 21):
2322 + 21 = 2343
But, this is still not the correct answer. To get the correct answer, we need to subtract 182 (since 43 x 27 = 1161, and 2343 - 1161 = 1182, then 1182 - 1161 = 21, and 21 x 2 = 42, 42 + 140 = 182):
2343 - 182 = 2161
However, this is not the correct answer either. To get the correct answer, we need to subtract 1000 (since 43 x 27 = 1161):
2161 - 1000 = 1161
📝 Note: Mental math strategies can be more challenging than other methods, but they're an excellent way to develop your mental math skills and improve your multiplication accuracy.
In conclusion, mastering 2 digit by 2 digit multiplication requires practice, patience, and persistence. By using a combination of the standard algorithm, the lattice method, the partial products method, the nines trick, and mental math strategies, you can develop a strong foundation in multiplication and improve your math skills.
What is the best method for multiplying 2 digit numbers?

+
The best method for multiplying 2 digit numbers depends on the individual’s learning style and preferences. However, the standard algorithm is widely used and is an excellent way to develop your multiplication skills.
How can I improve my mental math skills?

+
Improving your mental math skills requires practice, patience, and persistence. Try using mental math strategies, such as the “double and double” method, and practice multiplying numbers quickly and accurately.
What is the nines trick, and how does it work?

+
The nines trick is a clever way to multiply numbers that end in 9. It involves using the fact that 9 is equal to 10 - 1, and then using this fact to simplify the multiplication problem.
Related Terms:
- 2 digit multiplication worksheets free
- printable 2 digit by multiplication
- multiplication two digit by pdf
- multiplication 2 digits by worksheet
- 2 digit by multiplication twinkl
- multiplication 2 digit by pdf



