2.1 Practice: Patterns & Inductive Reasoning Answers Explained
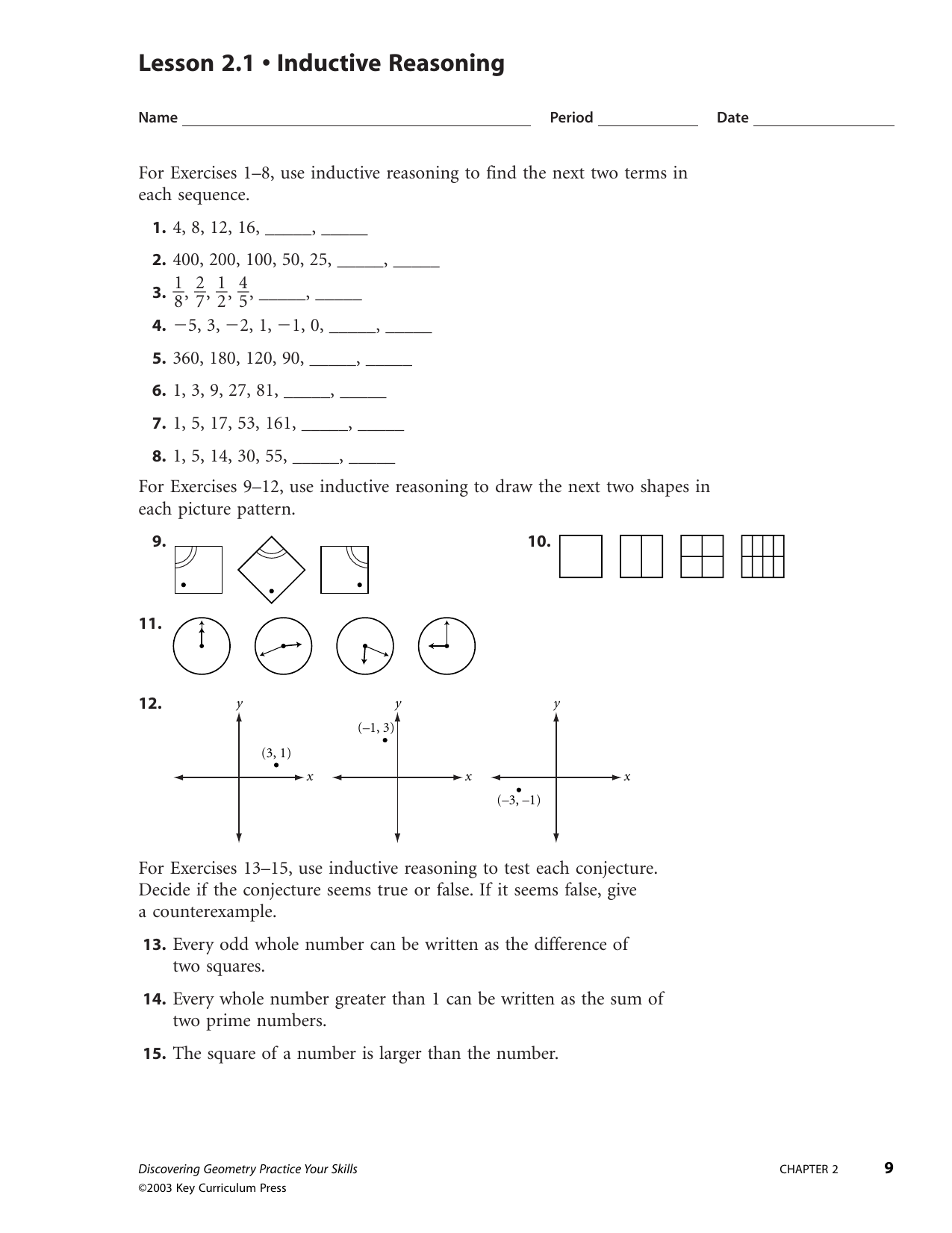
Understanding Patterns and Inductive Reasoning

Patterns are all around us; they structure our lives, our environments, and even our thought processes. In the world of mathematics, especially at educational levels where foundational skills are being established, understanding patterns and engaging in inductive reasoning are pivotal. This blog post delves into how these concepts work, why they’re important, and how you can master them through practical exercises.
The Fundamentals of Patterns

Patterns involve elements that repeat in a predictable way. Recognizing a pattern requires attention to detail and the ability to identify repetition or growth. Here are some foundational aspects of patterns:
- Repeating Patterns: These are sequences where elements repeat at fixed intervals. For example, in the sequence 1, 2, 3, 1, 2, 3…
- Growing Patterns: Elements increase or change systematically. An example might be the sequence where each number is double the last one: 2, 4, 8, 16…
- Functional Relationships: Patterns can also express relationships between quantities, where one variable changes as a function of another.
Inductive Reasoning: Drawing Generalizations from Patterns

Inductive reasoning is the process of making generalizations or predictions from specific observations. Here’s how it ties into pattern recognition:
- Observation: Identify a recurring theme or structure in a sequence.
- Hypothesis: Formulate a hypothesis about the behavior or the rule governing the pattern.
- Verification: Check the hypothesis against new or additional observations to refine or validate it.
Practical Application: Patterns in Math Education

Let’s look at a practical example often used in educational settings:
| Step | Description | Example |
|---|---|---|
| 1. Recognize | Identify the repeating or growing sequence. | 2, 4, 6, 8... |
| 2. Hypothesize | Formulate the rule. Here, each number increases by 2. | The rule might be n + 2 |
| 3. Apply | Use the rule to predict the next terms or find missing terms. | Next number: 8 + 2 = 10 |

💡 Note: Not all patterns are simple increments or decrements. Sometimes, they involve complex functions or changes.
Mastering Inductive Reasoning with Real-World Examples

Inductive reasoning isn’t just academic; it’s integral to daily problem-solving:
- Weather Predictions: If the morning dew is heavy and the wind is from the east, you might predict rain by observing this pattern over several days.
- Stock Market Analysis: Observing trends in stock prices can help in predicting future movements, although this is often subject to numerous external factors.
Exercises for Enhancing Pattern Recognition and Inductive Reasoning

Here are a few exercises you can try to bolster these skills:
- Number Sequences: Given a sequence like 1, 1, 2, 3, 5, 8, predict the next number.
- Shape Patterns: A sequence of shapes where each transformation follows a rule, like a square turning into a triangle.
- Data Analysis: Work with real data sets to predict future values or trends.
🌟 Note: While these exercises can sharpen your skills, they also train you to think critically and systematically.
Beyond Numbers: Patterns in Life and Art
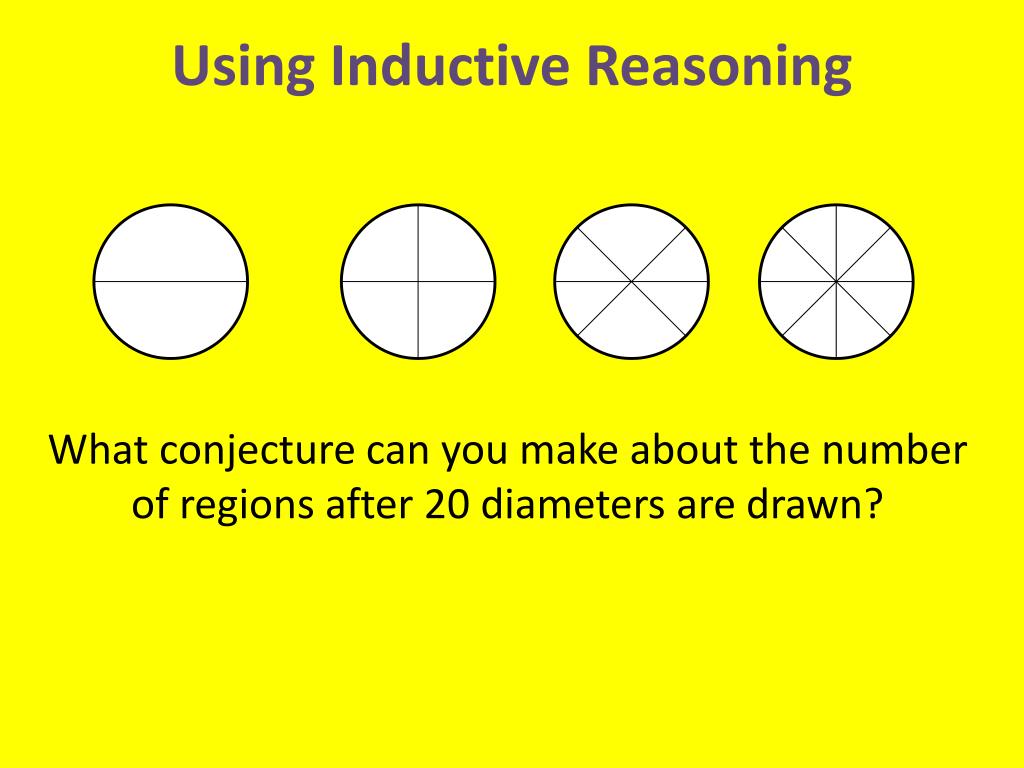
Patterns aren’t confined to numbers; they permeate:
- Art: Artists like M.C. Escher used patterns to create optical illusions and breathtaking designs.
- Nature: The Fibonacci Sequence in plant structures, like the arrangement of leaves or pinecones.
- Culture: Patterns in music, dance, storytelling, and more.
Final Insights on Patterns and Inductive Reasoning
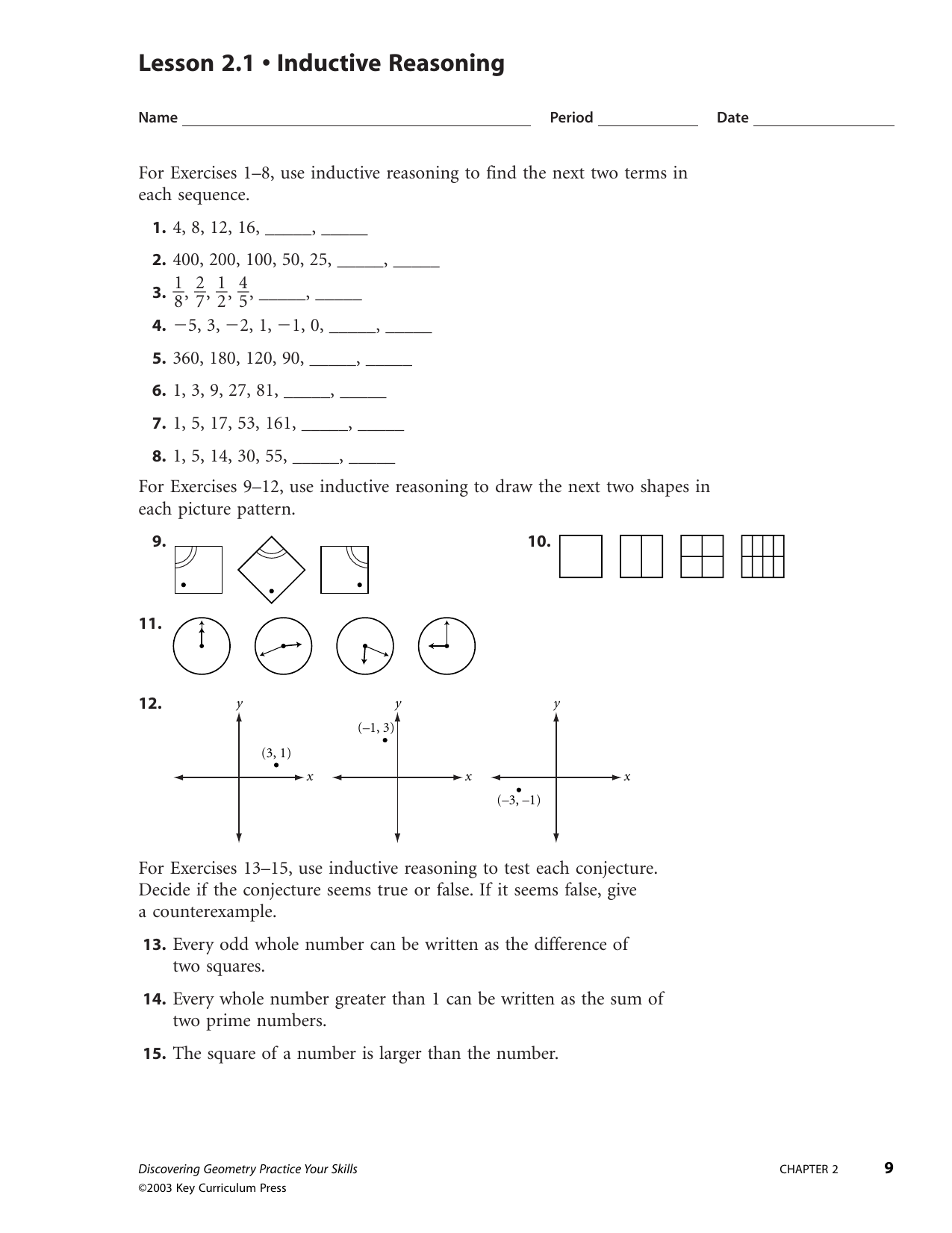
In the wrap-up, mastering patterns and inductive reasoning provides a solid foundation for logical thinking and problem-solving. From mathematics to everyday decisions, the ability to recognize, formulate hypotheses from, and predict based on patterns is crucial. Here are the key takeaways:
- Patterns can be simple or complex, mathematical or contextual.
- Inductive reasoning is about drawing conclusions from the observed patterns.
- Practical application of these skills can lead to better predictions and problem-solving capabilities.
How can pattern recognition improve problem-solving?

+
Recognizing patterns allows you to understand the underlying rules or relationships within problems, making it easier to find solutions. It helps in breaking down complex problems into manageable parts.
What is the difference between inductive and deductive reasoning?

+
Inductive reasoning starts with specific observations and forms general conclusions, whereas deductive reasoning starts with general statements or hypotheses and tests specific cases to validate them.
Can you give an example of a pattern in daily life?

+
Yes! If you notice that your commute time increases by 5 minutes every time there’s a reported construction delay on your route, that’s a recognizable pattern that could help you plan your journey better in the future.